A new model of wave energy dissipation for multiple wave breaking on very mild beach
-
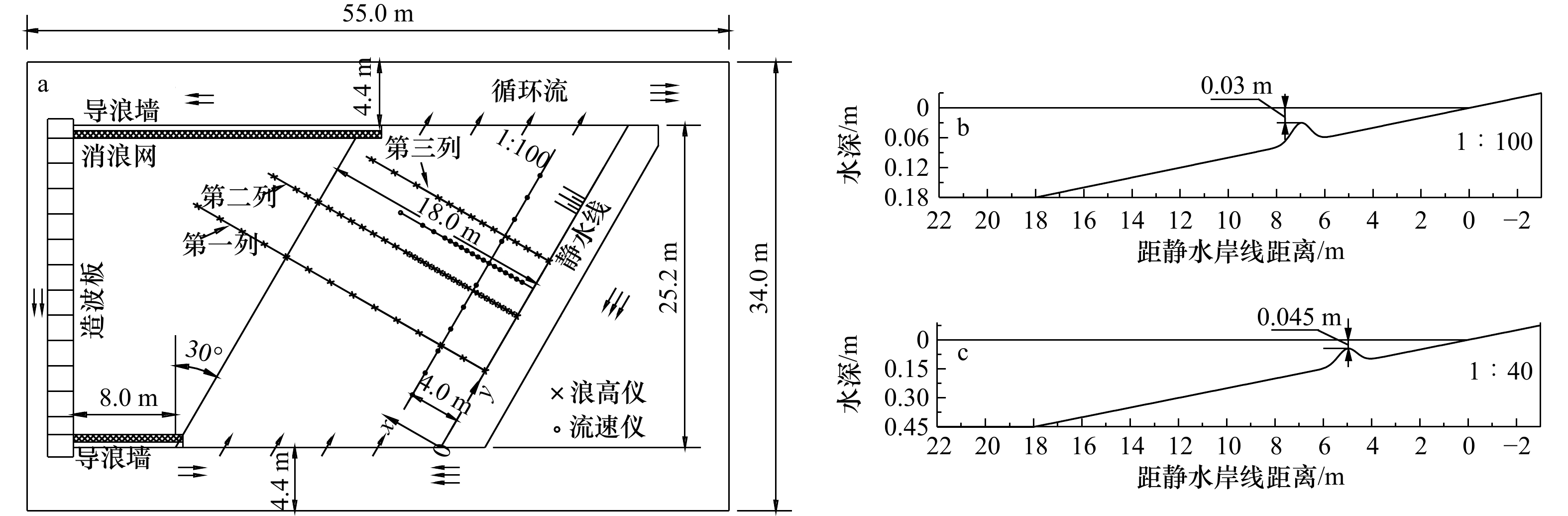
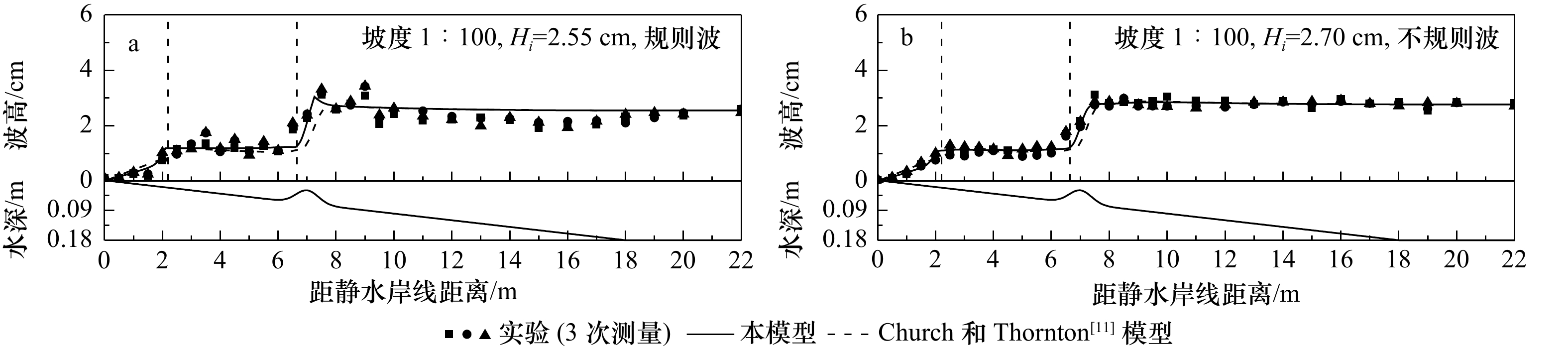
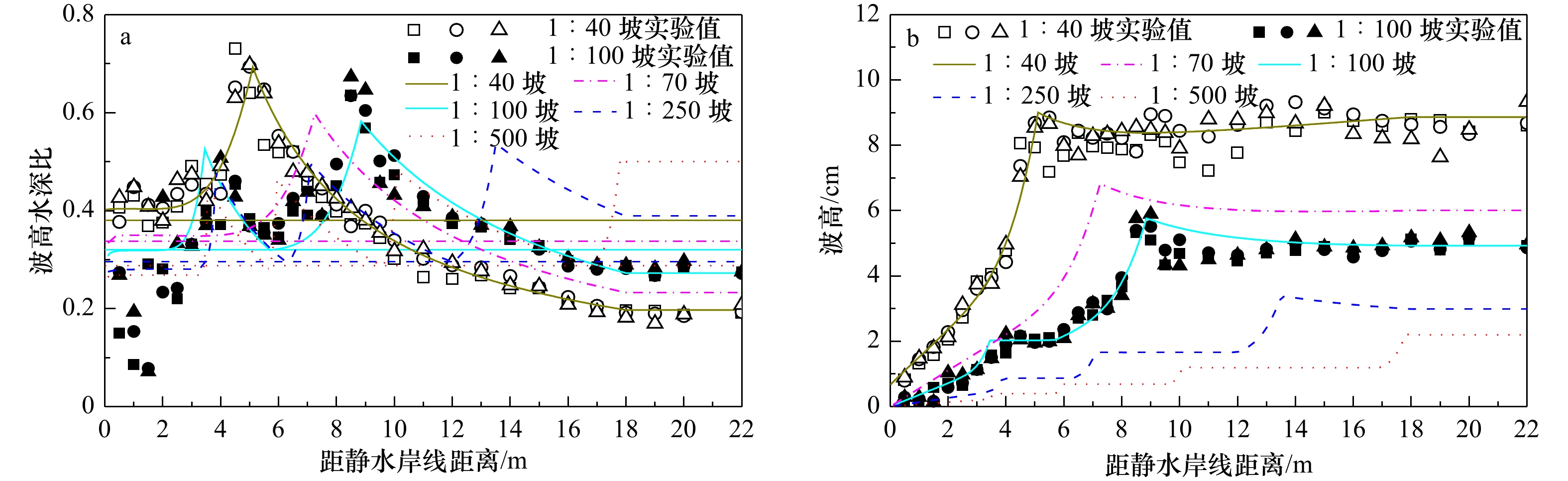
摘要: 在坡度很缓(接近或小于1∶100)的海岸，波浪在向海岸传播的过程中，可能经历多次破碎，而在两次波浪破碎之间将伴随着波浪恢复(波浪恢复到不破碎状态)。在现有海岸波高计算模型中，波浪破碎是通过波能耗散来模拟的，但所采用的波能耗散模型都不能自动考虑波浪出现多次破碎的过程，特别精确模拟这一过程中出现的波浪恢复。本文提出了解决这一问题的新的波能耗散模型，模型的建立是通过在Dally模型中重新建立稳定波能、饱和波高水深比和波能耗散系数，并引入了波浪恢复的判断条件实现的。该模型的波能耗散在波浪恢复区的值很小故能描述波浪恢复区的波浪运动。与实验结果的对比表明，新模型可以适合缓坡情况波浪多次破碎的波高模拟，而且对不同坡度的平坡和沙坝海岸(1∶100～1∶10)的破碎波模拟都可以给出与实验结果符合的结果，并且可以自动识别多次波浪破碎的存在和波浪恢复的发生。Abstract: On a coastal beach of very mild slope (close to or less than 1∶100), the multiple wave breaking may occur when waves propagate to the coast, companied by the wave recovery process between two breaking. In the current models for calculation of wave height, the wave breaking is usually simulated by the wave energy dissipation. But the establishment of energy dissipation describing wave breaking effect in these models did not automatically take the multiple wave breakings into account, and especially did not accurately simulate the wave recovery in this process. The present study presents a new model of the wave energy dissipation which can overcome this problem. The model is established by re-determining the stable wave energy, the saturated wave height-depth ratio and the dissipation coefficient and by introducing the judgment condition for the occurrence of wave recovery. The model has a very small value of the energy dissipation over wave recovery region and can properly describe the wave motion in wave recovery process. Comparison with measured wave heights shows that the model can give the wave heights on the plane and barred beaches of various bottom slopes (1∶10 to 1∶100), which agree well with the measurement, especially the multiple wave breaking and wave recovery process can be recognized automatically in the numerical simulations.
-
Key words:
- wave recovery /
- wave breaking /
- wave energy dissipation /
- mild plane beach /
- barred beach
-
图 1 不同海岸坡度情况波高水深比（a）和波高（b）在垂直海岸方向的变化
Fig. 1 The cross-shore variations of wave height-depth ratios (a) and wave heights (b) calculated by the present model for different typical slopes
图 2 实验布置平面图（1∶100平坡海岸，1∶40坡的与此相似）（a）和沙坝海岸横剖面（b，c）
Fig. 2 The plan view of experimental layout (1∶100 plane beach, similar for the slope 1∶40)(a) and the vertical profiles of barred beaches (b, c)
图 3 平坡海岸波高的计算结果和实验结果的对比(规则波情况）
两条竖虚线分别代表第一次波浪的结束和第二次破碎的开始
Fig. 3 Comparison of computed and measured cross-shore wave heights for regular waves
Two vertical dashed lines represent the end of the first wave breaking and the beginning of the second wave breaking
图 4 沙坝海岸规则波（a，c）和不规则波（b，d）的波高和波能耗散空间变化形态（坡度1∶40）
Fig. 4 Cross-shore variations of wave height and energy dissipation on barred beach with slope 1∶40 for regular wave (a, c) and irregular wave (b, d)
图 5 沙坝海岸规则波（a）和不规则波（b）的波高空间变化（坡度1∶100）
Fig. 5 Cross-shore variations of wave height on barred beach with slope 1∶100 for regular wave (a) and irregular wave (b)
-
[1] Battjes J A, Janssen J P F M. Energy loss and set-up due to breaking of random waves[C]//Proceeding of Coastal Engineering Conference. Hamburg, Germany: ASCE, 1978: 569−587. [2] Dally W R, Dean R G, Dalrymple R A. Wave height variation across beaches of arbitrary profile[J]. Journal of Geophysical Research: Oceans, 1985, 90(C6): 11917−11927. doi: 10.1029/JC090iC06p11917 [3] Roelvink J A. Dissipation in random wave groups incident on a beach[J]. Coastal Engineering, 1993, 19(1/2): 127−150. [4] Zhao L, Panchang V, Chen W, et al. Simulation of wave breaking effects in two-dimensional elliptic harbor wave models[J]. Coastal Engineering, 2001, 42(4): 359−373. doi: 10.1016/S0378-3839(00)00069-7 [5] Daly C, Roelvink D, van Dongeren A, et al. Validation of an advective-deterministic approach to short wave breaking in a surf-beat model[J]. Coastal Engineering, 2012, 60: 69−83. doi: 10.1016/j.coastaleng.2011.08.001 [6] Tajima Y, Madsen O S. Modeling near-shore waves, surface rollers, and undertow velocity profiles[J]. Journal of Waterway, Port, Coastal, and Ocean Engineering, 2006, 132(6): 429−438. doi: 10.1061/(ASCE)0733-950X(2006)132:6(429) [7] 沈良朵. 缓坡沿岸流不稳定实验研究和计算分析[D]. 大连: 大连理工大学, 2014.Shen Liangduo.Study of the feature of longshore current and its instability on mild beach slope[D]. Dalian: Dalian University of Technology, 2014. [8] 王彦.沙坝海岸沿岸流和裂流特征研究[D]. 大连: 大连理工大学, 2017.Wang Yan. Study on the longshore currents and rip currents on barred beaches[D]. Dalian: Dalian University of Technology, 2017. [9] Ruessink B G, Miles J R, Feddersen F, et al. Modeling the alongshore current on barred beaches[J]. Journal of Geophysical Research: Oceans, 2001, 106(C10): 22451−22463. doi: 10.1029/2000JC000766 [10] Visser P J. Laboratory measurements of uniform longshore currents[J]. Coastal Engineering, 1991, 15(5/6): 563−593. [11] Church J C, Thornton E B. Effects of breaking wave induced turbulence within a longshore current model[J]. Coastal Engineering, 1993, 20(1/2): 1−28. -
下载:
计量
- 文章访问数: 265
- HTML全文浏览量: 77
- PDF下载量: 16
- 被引次数: 0
