机载GNSS反射信号海面测高模型的研究

张云; 张杨阳; 孟婉婷; 杨树瑚; 韩彦岭

doi:10.3969/j.issn.0253-4193.2020.03.015

机载GNSS反射信号海面测高模型的研究

doi: 10.3969/j.issn.0253-4193.2020.03.015

1.
上海海洋大学信息学院，上海 201306
2.
上海航天电子技术研究所，上海 201109

基金项目: 国家自然科学基金（41376178，41401489）；上海市大学青年教师基金（ZZHY13033）。

详细信息

作者简介:
张云（1974—）男，上海市人，教授，主要从事卫星导航定位和GNSS-R技术研究。E-mail：y-zhang@shou.edu.cn

中图分类号: P228.3
计量
- 文章访问数: 452
- HTML全文浏览量: 139
- PDF下载量: 69
- 被引次数: 0
出版历程
- 收稿日期: 2019-02-22
- 修回日期: 2019-09-06
- 网络出版日期: 2020-11-18
- 刊出日期: 2020-03-25

Research on sea surface altimetry model of airborne GNSS reflected signal

1.
College of Information, Shanghai Ocean University, Shanghai 201306, China
2.
Shanghai Aerospace Electronic Technology Institute, Shanghai 201109, China

摘要

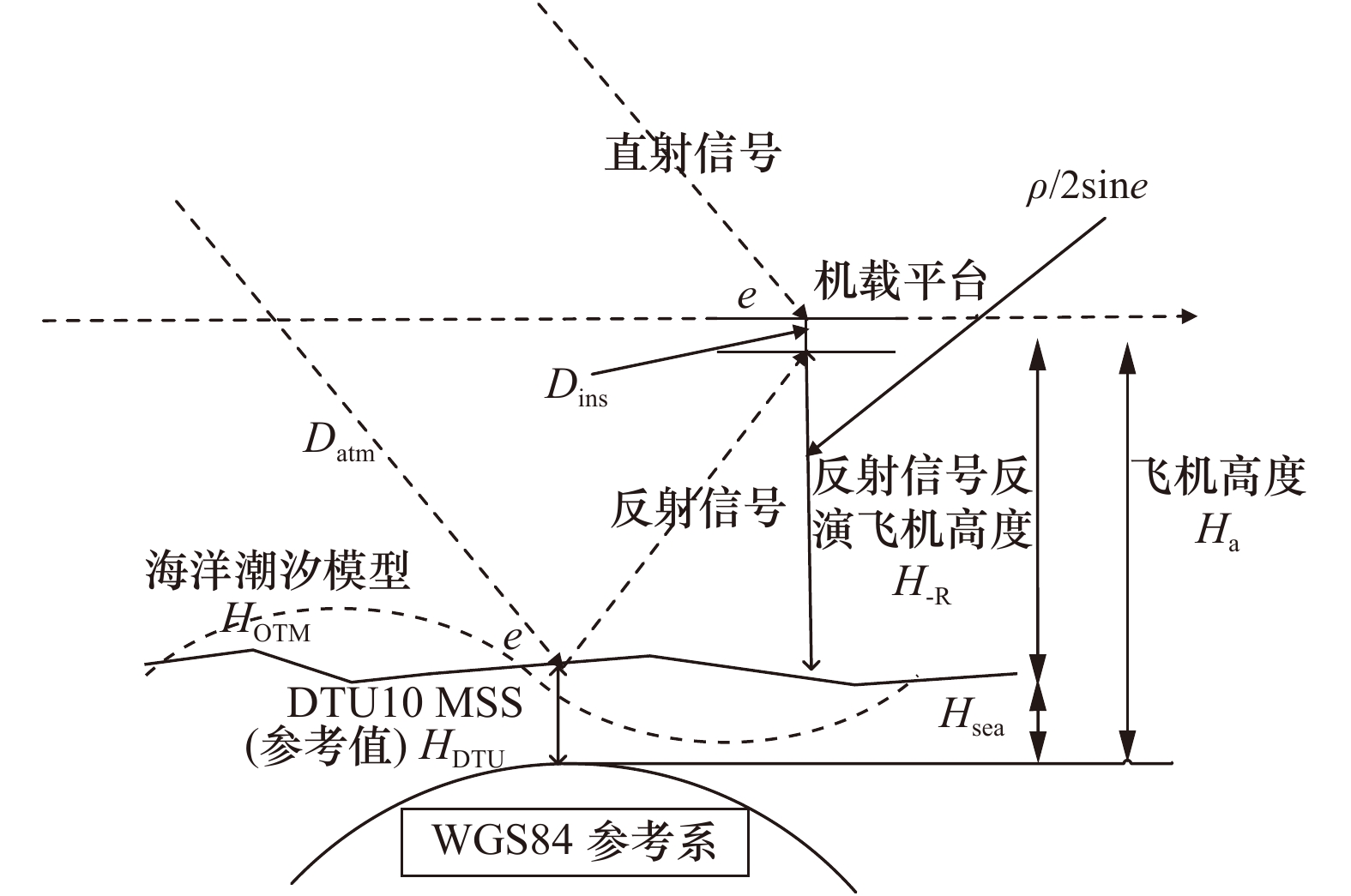
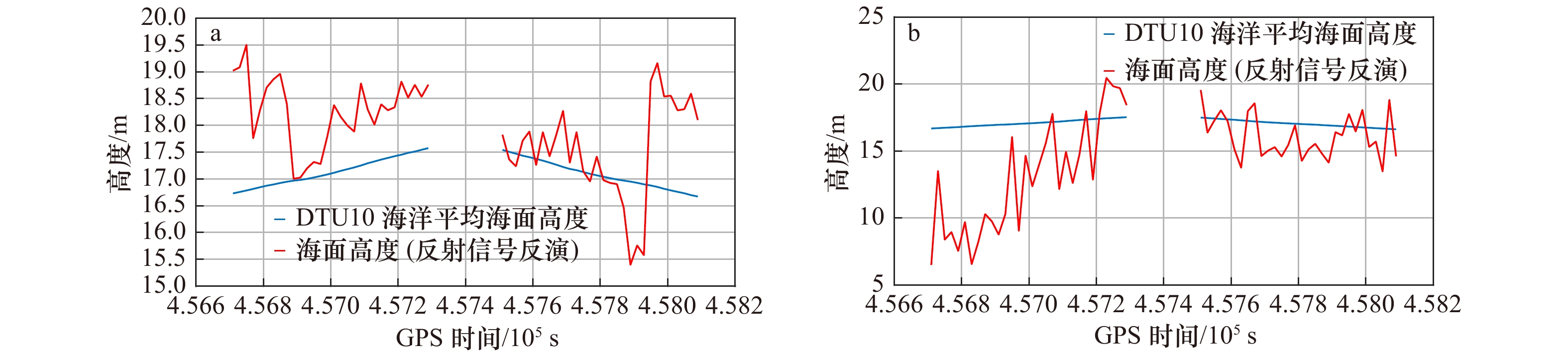
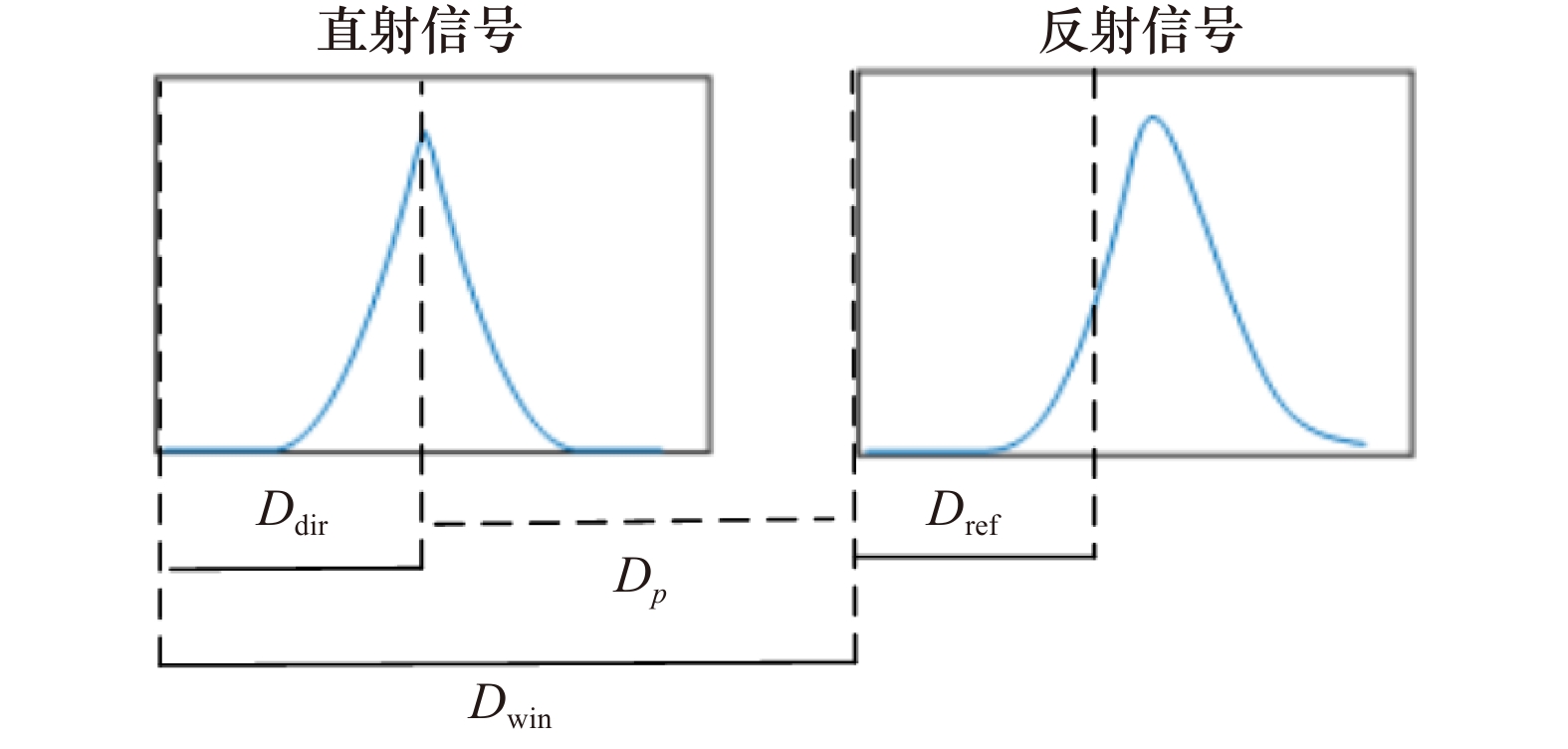
摘要: 相对于岸基GNSS-R技术，机载GNSS-R优势在于其空间分辨率高、监测范围广，可对特定区域范围进行高分辨率监测，兼具了灵活的高度和方位调节的同时保障了更高的数据质量。本文主要研究了机载GNSS-R测高模型，依据岸基GNSS-R码测高原理，针对大气延迟、天线距离等进行修正，优化机载测高模型，同时采用DTU10全球海面平均高度及潮汐模型验证机载GNSS-R测高模型的精度。通过分析2011年11月11日，CSIC-IEEC在芬兰波罗的海的GNSS-R机载数据，针对不同仰角下的实验数据进行反演，成功地实现了亚米级机载海面高度反演，得出仰角大小会对测高结果精度产生较大影响的结论，定性分析了仰角大小所引起的误差范围。本文的结果证明了机载GNSS-R的海面测高的可行性。
- GNSS-R /
- 机载实验 /
- 海面测高 /
- 精度分析
Abstract: Compared with shore-based GNSS-R technology, the airborne GNSS-R has the advantages of high spatial resolution, wide monitoring range, high-resolution monitoring of specific areas, and flexible height and azimuth adjustments. This paper mainly studies the airborne GNSS-R altimetry model, based on the shore-based GNSS-R code altimetry principle, corrects the atmospheric delay, antenna distance, etc., optimizes the airborne altimetry model, and uses the DTU10 global sea level average height and tide. The model verifies the accuracy of the onboard GNSS-R altimetry model. By analyzing the GNSS-R airborne data of the CSIC-IEEC in the Baltic Sea in Finland on November 11, 2011, the inversion of the experimental data at different elevation angles was successfully carried out, and the inversion of the sub-meter airborne sea surface height was successfully achieved. The conclusion that the elevation angle has a great influence on the accuracy of the altimetry results qualitatively analyzes the error range caused by the elevation angle. The results of this paper demonstrate the feasibility of sea level altimetry for airborne GNSS-R.
- GNSS-R /
- airborne experiment /
- sea surface altimetry /
- accuracy analysis

HTML全文

图 1 GNSS-R码测高技术基本概念

Fig. 1 GNSS-R group delay altimetry basic concept

下载: 全尺寸图片幻灯片

图 2 机载测高模型

Fig. 2 Airborne altimetry model

下载: 全尺寸图片幻灯片

图 3 飞行轨迹图

a. 第一个实验期间飞行轨迹；b. 第二个实验期间飞行轨迹

Fig. 3 Flight trajectory

a is the flight trajectory during the first experiment; b is the flight trajectory during the second experiment

下载: 全尺寸图片幻灯片

图 4 PRN12号卫星（红）及PRN25号卫星（蓝）仰角变化

a. 第一个实验期间仰角变化；b. 第二个实验期间仰角变化

Fig. 4 Variation of PRN12 satellite (red) and PRN25 satellite (blue) elevation angle

a is the elevation angle change during the first experiment; b is the elevation angle change during the second experiment

下载: 全尺寸图片幻灯片

图 5 PRN12（红色）和PRN25（蓝色）卫星镜面反射点轨迹

a. 第一个实验期间镜面反射点轨迹；b. 第二个实验期间镜面反射点轨迹

Fig. 5 PRN12 (red) and PRN25 (blue) satallite specular reflection point trace

a is the specular reflection point trajectory during the first experiment; b is the specular reflection point trajectory during the second experiment

下载: 全尺寸图片幻灯片

图 6 第一个数据时间段1 s高度对比

a. 高仰角情况下高度对比；b. 低仰角情况下高度对比

Fig. 6 The first data period 1 s height comparison

a is height comparison for high elevation angles; b is height comparison for low elevation angles

下载: 全尺寸图片幻灯片

图 7 第一个数据时间段20 s平均后高度对比

a. 高仰角情况下高度对比；b. 低仰角情况下高度对比

Fig. 7 The first data period after 20 s average height comparison

a is height comparison for high elevation angles; b is height comparison for low elevation angles

下载: 全尺寸图片幻灯片

图 8 反演高度异常值（a）及筛选过后高度值（b）

Fig. 8 Inversion of height anomaly values（a）and filtered height values（b）

下载: 全尺寸图片幻灯片

图 9 直射信号正常功率波形（a）及异常功率波形（b）

Fig. 9 Direct signal normal（a）and abnormal power waveform（b）

下载: 全尺寸图片幻灯片

图 10 第二个数据时间段1 s高度对比

a. 高仰角情况下高度对比；b.低仰角情况下高度对比

Fig. 10 The second data period 1 s height comparison

a is height comparison for high elevation angles; b is height comparison for low elevation angles

下载: 全尺寸图片幻灯片

图 11 第二个数据时间段20 s平均后高度对比

a.高仰角情况下高度对比；b.低仰角情况下高度对比

Fig. 11 The second data period after 20 s average height comparison

a is height comparison for high elevation angles; b is height comparison for low elevation angles

下载: 全尺寸图片幻灯片

表 1 实验数据结果

Tab. 1 Result of experimental data

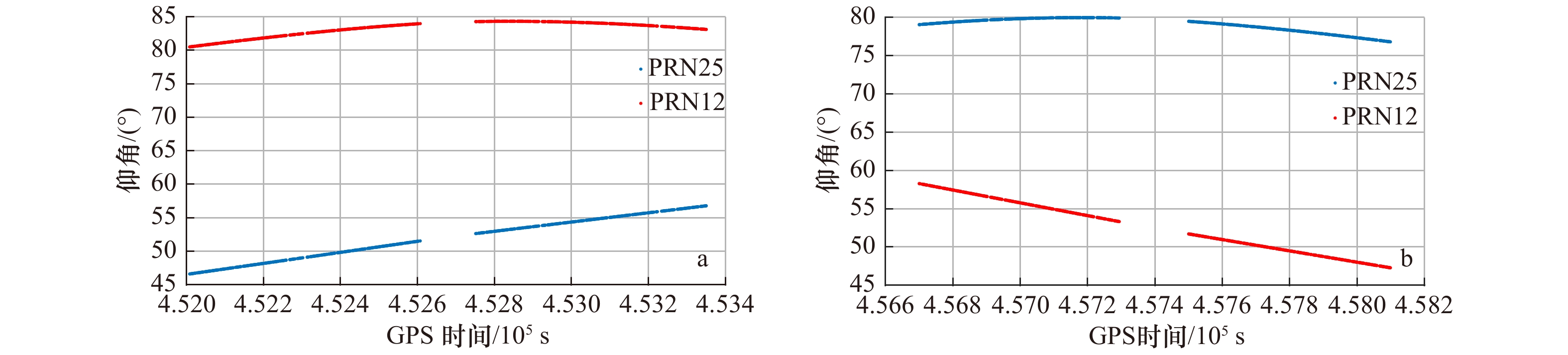
数据	GPS时间	仰角	卫星号	低/高仰角
第一个数据时间段	452 008～453 350 s	80.51°～84.29°	12	高
第一个数据时间段	452 008～453 350 s	46.60°～56.78°	25	低
第二个数据时间段	456 700～458 099 s	76.82°～79.93°	25	高
第二个数据时间段	456 700～458 099 s	47.30°～58.30°	12	低

下载: 导出CSV

表 2 实验数据结果

Tab. 2 Result of experimental data

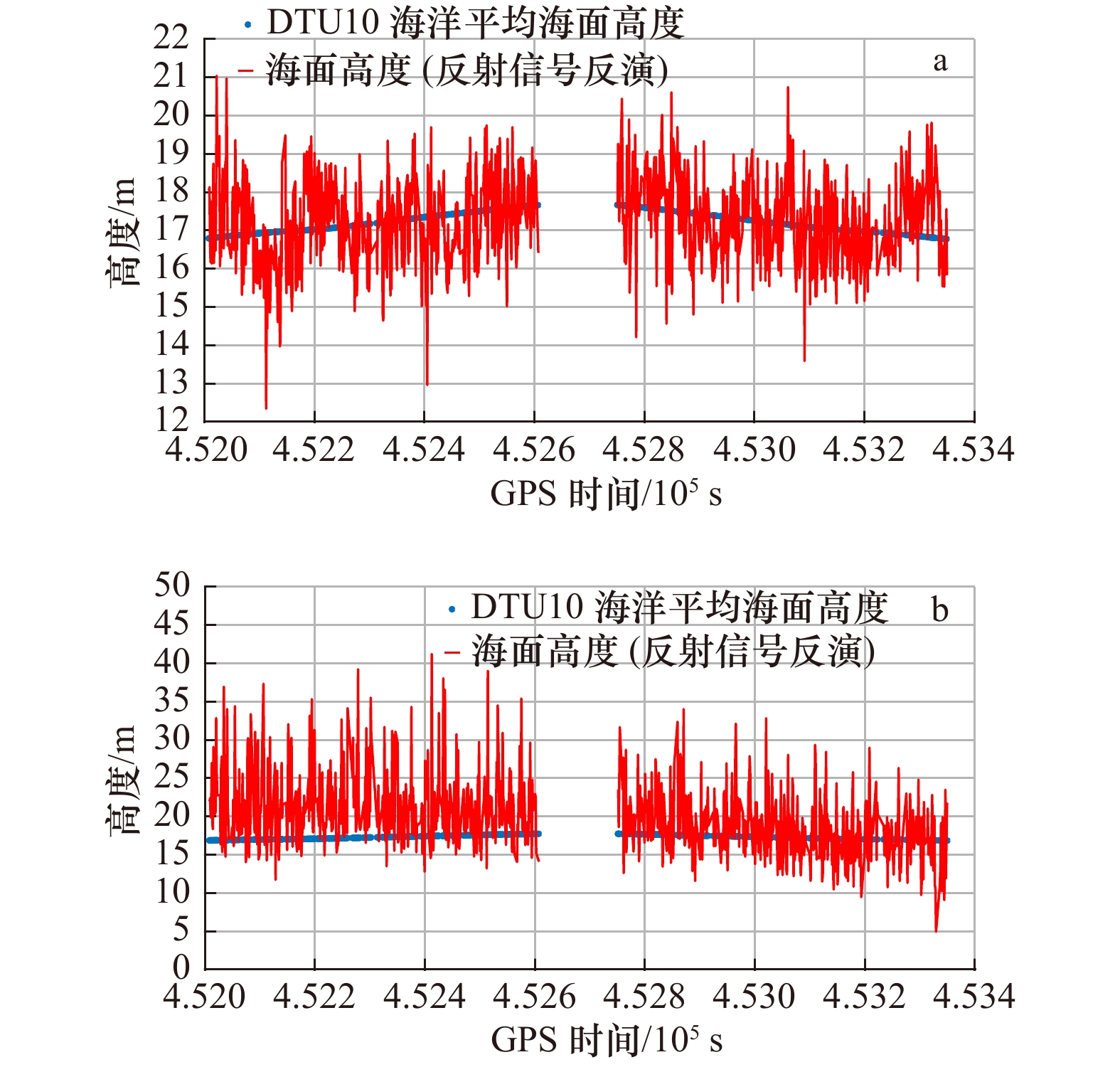
实验数据	卫星号	仰角	评估标准	1 s	10 s	15 s	20 s
第一个数据时间段	12	高	Bias/m	0.009	0.012	−0.006	−0.007
			MAE/m	0.890	0.492	0.462	0.447
			STD/m	0.690	0.395	0.347	0.329
	25	低	Bias/m	2.600	2.595	2.581	2.578
			MAE/m	3.934	2.922	2.879	2.811
			STD/m	3.781	2.078	1.866	1.789
第二个数据时间段	25	高	Bias/m	0.798	0.792	0.789	0.792
			MAE/m	1.211	1.002	0.981	0.972
			STD/m	0.874	0.727	0.721	0.718
	12	低	Bias/m	−2.362	−2.544	−2.543	−2.533
			MAE/m	5.900	3.523	3.226	3.179
			STD/m	5.056	3.341	2.951	2.818
注：Bias表示偏差，MAE表示平均绝对误差，STD表示平均绝对误差的标准偏差。

下载: 导出CSV

参考文献(28)

[1]	孙剑. GNSS-R海洋反射接收机的控制设计及实现[D]. 北京: 中国科学院空间科学与应用研究中心, 2010. Sun Jian. Design and realization of control system for the GNSS-R receiver[D]. Beijing: Center for Space Science and Applier Research Chinese Academy of Sciences, 2010.
[2]	Martin-Neira M. A passive reflectometry and interferometry system (PARIS): application to ocean altimetry[J]. ESA Journal, 1993, 17(4): 331−355.
[3]	Park H, Valencia E, Camps A, et al. Delay tracking in spaceborne GNSS-R ocean altimetry[J]. IEEE Geoscience and Remote Sensing Letters, 2013, 10(1): 57−61. doi: 10.1109/LGRS.2012.2192255
[4]	Ablain M, Cazenave A, Valladeau G, et al. A new assessment of the error budget of global mean sea level rate estimated by satellite altimetry over 1993-2008[J]. Ocean Science, 2009, 5(2): 193−201. doi: 10.5194/os-5-193-2009
[5]	Cardellach E, Nogues-Correig O, Ribo S, et al. Centimeter-level group-delay altimetric precision using the new PARIS interferometric technique[C]//American Geophysical Union, Fall Meeting 2010. AGU, 2010.
[6]	Berry R, Mattos P G, Kale I. Group delay and phase delay in GNSS systems[J]. Geo-spatial Information Science, 2013, 16(3): 210−219. doi: 10.1080/10095020.2013.834111
[7]	Guo Fei, Zhang Xiaohong, Wang Jinling. Timing group delay and differential code bias corrections for BeiDou positioning[J]. Journal of Geodesy, 2015, 89(5): 427−445. doi: 10.1007/s00190-015-0788-2
[8]	Semmling A M, Leister V, Saynisch J, et al. A phase-altimetric simulator: studying the sensitivity of earth-reflected GNSS signals to ocean topography[J]. IEEE Transactions on Geoscience and Remote Sensing, 2016, 54(11): 6791−6802. doi: 10.1109/TGRS.2016.2591065
[9]	Cardellach E, Ao C O, De la Torre Juárez M, et al. Carrier phase delay altimetry with GPS-reflection/occultation interferometry from low Earth orbiters[J]. Geophysical Research Letters, 2004, 31(10): L10402.
[10]	Germain O, Ruffini G. A revisit to the GNSS-R code range precision[C]//Proceedings of the GNSS-R’06 Workshop. The Netherlands, 2006.
[11]	Stosius R, Beyerle G, Semmling M, et al. Tsunami detection from space using GNSS Reflections: Results and activities from GFZ[C]//2010 IEEE International Geoscience and Remote Sensing Symposium. Honolulu, HI, USA: IEEE, 2010.
[12]	Carreno-Luengo H, Camps A, Ramos-Pérez I, et al. Experimental evaluation of GNSS-reflectometry altimetric precision using the P(Y) and C/A signals[J]. IEEE Journal of Selected Topics in Applied Earth Observations and Remote Sensing, 2014, 7(5): 1493−1500. doi: 10.1109/JSTARS.2014.2320298
[13]	Carreno-Luengo H, Camps A, Perez-Ramos I, et al. 3Cat-2: A P(Y) and C/A GNSS-R experimental nano-satellite mission[C]//IEEE International Geoscience and Remote Sensing Symposium. Melbourne, VIC, Australia: IEEE, 2014.
[14]	Rius A, Fabra F, Ribó S, et al. PARIS Interferometric Technique proof of concept: Sea surface altimetry measurements[C]//2012 IEEE International Geoscience and Remote Sensing Symposium. Munich, Germany: IEEE, 2012.
[15]	Park J, Johnson J T, O'Brien A, et al. Studies of TDS-1 GNSS-R ocean altimetry using a “full DDM” retrieval approach[C]//2016 IEEE International Geoscience and Remote Sensing Symposium. Beijing, China: IEEE, 2016.
[16]	Mashburn J, Axelrad P, Lowe S T, et al. Global ocean altimetry with GNSS reflections from TechDemoSat-1[J]. IEEE Transactions on Geoscience and Remote Sensing, 2018, 56(7): 4088−4097. doi: 10.1109/TGRS.2018.2823316
[17]	Zhang Yun, Li Binbin, Tian Luman, et al. Phase altimetry using reflected signals from BeiDou GEO satellites[J]. IEEE Geoscience and Remote Sensing Letters, 2016, 13(10): 1410−1414. doi: 10.1109/LGRS.2016.2578361
[18]	Larson K M, Ray R D, Nievinski F G, et al. The accidental tide gauge: a GPS reflection case study from Kachemak bay, Alaska[J]. IEEE Geoscience and Remote Sensing Letters, 2013, 10(5): 1200−1204. doi: 10.1109/LGRS.2012.2236075
[19]	Cardellach E, Rius A, Martín-Neira M, et al. Consolidating the precision of interferometric GNSS-R ocean altimetry using airborne experimental data[J]. IEEE Transactions on Geoscience and Remote Sensing, 2014, 52(8): 4992−5004. doi: 10.1109/TGRS.2013.2286257
[20]	Semmling A M, Beckheinrich J, Wickert J, et al. Sea surface topography retrieved from GNSS reflectometry phase data of the GEOHALO flight mission[J]. Geophysical Research Letters, 2014, 41(3): 954−960. doi: 10.1002/2013GL058725
[21]	Zhang Yun, Tian Luman, Meng Wanting, et al. Feasibility of code-level altimetry using coastal BeiDou reflection (BeiDou-R) setups[J]. IEEE Journal of Selected Topics in Applied Earth Observations and Remote Sensing, 2015, 8(8): 4130−4140. doi: 10.1109/JSTARS.2015.2446684
[22]	沈思明, 张云, 杨树瑚, 等. GNSS反射信号海面高度测量及风速对反演精度的影响分析[J]. 全球定位系统, 2017, 42(4): 83−89, 101. Shen Siming, Zhang Yun, Yang Shuhu, et al. Sea surface altimetry using GNSS-R signal and analysis of retrieval accuracy influenced by wind speed[J]. Gnss World of China, 2017, 42(4): 83−89, 101.
[23]	Alonso-Arroyo A, Querol J, Lopez-Martinez C, et al. SNR and standard deviation of cGNSS-R and iGNSS-R scatterometric measurements[J]. Sensors, 2017, 17(12): 183. doi: 10.3390/s17010183
[24]	Niell A E. Global mapping functions for the atmosphere delay at radio wavelengths[J]. Journal of Geophysical Research: Solid Earth, 1996, 101(B2): 3227−3246. doi: 10.1029/95JB03048
[25]	Stammer D, Ray R D, Andersen O B, et al. Accuracy assessment of global barotropic ocean tide models[J]. Reviews of Geophysics, 2014, 52(3): 243−282. doi: 10.1002/2014RG000450
[26]	Haddad M, Hachemi H, Taibi H. Assessment of Gravity Anomaly Surfaces (DTU10, EGM2008 and ITG-Goce02) in Western Mediterranean Sea[J]. Mediterranean Journal of Modeling and Simulation, 2015, 3(1): 87−99.
[27]	Clarizia M P, Ruf C, Cipollini P, et al. First spaceborne observation of sea surface height using GPS-Reflectometry[J]. Geophysical Research Letters, 2016, 43(2): 767−774. doi: 10.1002/2015GL066624
[28]	Li Weiqiang, Cardellach E, Fabra F, et al. First spaceborne phase altimetry over sea ice using TechDemoSat-1 GNSS-R signals[J]. Geophysical Research Letters, 2017, 44(16): 8369−8376. doi: 10.1002/2017GL074513