短峰畸形波生成、演化过程的外部特征研究

崔成; 潘文博

doi:10.12284/hyxb2023093

短峰畸形波生成、演化过程的外部特征研究

doi: 10.12284/hyxb2023093

崔成^1,,
潘文博¹

交通运输部天津水运工程科学研究所港口水工建筑技术国家工程研究中心，天津 300456

基金项目: 国家自然科学基金青年项目（51509120）；国家自然科学基金面上项目（52171260）；中央级公益性科研院所基本科研基金（TKS20200317）

详细信息

作者简介:
崔成（1984-），男，辽宁省海城市人，主要从事随机波浪及其与结构物相互作用研究。 E-mail：chengcui1984@163.com

中图分类号: P731.22
计量
- 文章访问数: 217
- HTML全文浏览量: 113
- PDF下载量: 30
- 被引次数: 0
出版历程
- 收稿日期: 2022-12-09
- 修回日期: 2023-01-30
- 网络出版日期: 2023-07-26
- 刊出日期: 2023-07-01

Study on the external characteristics of the generation and evolution of short-crested freak waves

Cui Cheng^1
,,
Pan Wenbo¹

National Engineering Research Center of Port Hydraulic Construction Technology, Tianjin Research Institute of Water Transport Engineering, Ministry of Transport, Tianjin 300456, China

摘要

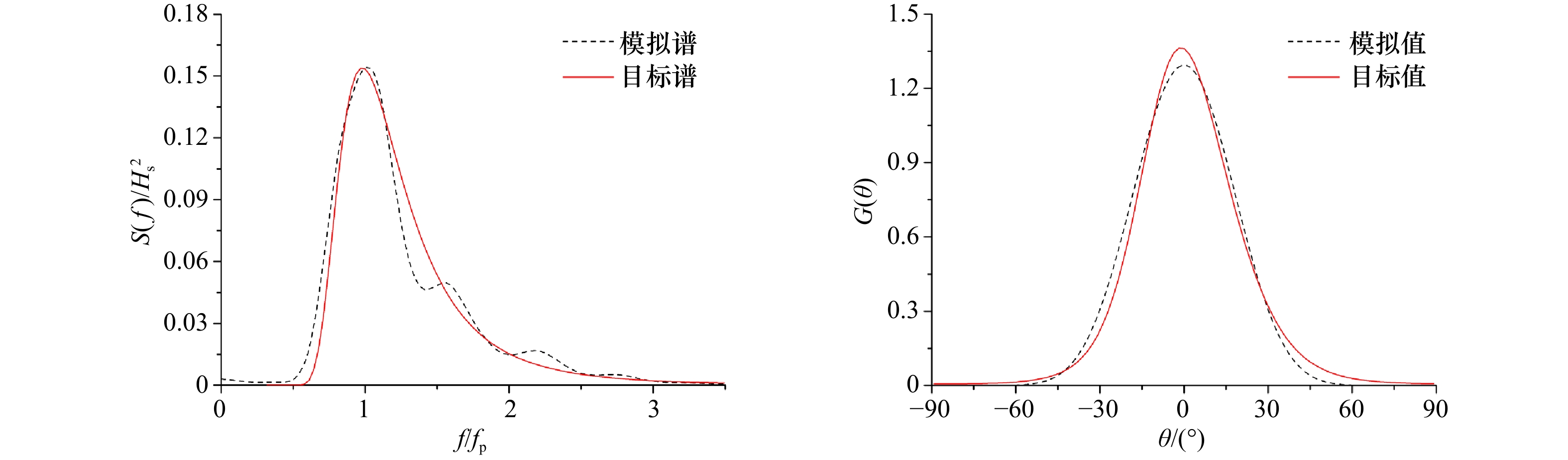
摘要: 在实际海况中，由于波浪组分方向不同，使海浪常表现为三维短峰波，与二维长峰波相比，有诸多异同特征。为了研究三维畸形波生成和演化过程中异常大波的外部特征以及大波间的关联，本文采用色散和方向聚焦方法在物理水池中模拟了短峰畸形波的整个生成和演化过程。研究结果表明，生成和演化过程中出现的异常大波沿着方向分布函数峰值方向演化，具有一定程度“对称”特征；根据异常大波的特征参数可将该过程分成3～4个典型的阶段，这意味着可以根据异常大波的特征参数判断其所处阶段及预测其演化趋势；三维畸形波生成和演化过程可能会跳过“波群”或者“深谷”阶段，与二维畸形波相比，时−空范围较小，这意味着在三维波浪场中，与畸形波相关的深谷和波群的发生概率较低。
- 畸形波 /
- 生成和演化 /
- 三维 /
- 多向波 /
- 短峰波
Abstract: In the real sea state, the differences in directions of component waves result in three-dimensional short-crested waves, which have many different characteristics compared to two-dimensional long-crested waves. Therefore, in present study, the whole generation and evolution processes of short-crested freak waves are experimentally simulated by dispersive and directional focusing of component waves, in order to examine the external features and relationship of the abnormal large waves occurring during the processes. The results indicate that the abnormal large wave evolves symmetrically along the peak wave direction and undergoes a three- or four-stage process, each stage is indicated by the characteristic parameters of the abnormal large wave, it implies that the characteristic parameters can be used to identify the stage which the large abnormal wave is in and predict the variation tendency; and that compared to the two-dimensional cases, the “wave group” and “deep trough” stages are likely to be skipped, as a result, the spatio-temporal spans of the generation and evolution processes are smaller for the three-dimensional cases, it implies that in three-dimensional wave fields, the deep troughs and successive large waves (wave group) associated with freak waves may have a low probability of occurrence.
- freak wave /
- generation and evolution /
- three-dimension /
- multidirectional wave /
- short-crested wave

HTML全文

图 1 二维试验水槽和测波杆布置图

Fig. 1 The sketch of the wave flume and wave gauge locations for two-dimension experiments

下载: 全尺寸图片幻灯片

图 2 三维试验水池和测波杆布置图

Fig. 2 The sketch of the wave basin and wave gauge locations for three-dimension experiments

下载: 全尺寸图片幻灯片

图 3 二维畸形波模拟谱和目标谱对比

Fig. 3 Comparison of target and simulated spectrum for the 2D freak wave

下载: 全尺寸图片幻灯片

图 4 二维畸形波生成、演化过程中异常大波无量纲波面

Fig. 4 Normalized surface elevations of abnormal large waves occurring during the generation and evolution process of a two-dimensional freak wave

下载: 全尺寸图片幻灯片

图 5 三维畸形波模拟谱和目标谱对比

Fig. 5 Comparison of target and simulated spectrum for the 3D freak wave

下载: 全尺寸图片幻灯片

图 6 工况1三维畸形波生成、演化过程中异常大波无量纲波面时间过程

Fig. 6 Normalized surface elevations of abnormal large waves occurring during the generation and evolution process of the three-dimensional freak wave for Case 1

下载: 全尺寸图片幻灯片

图 7 工况2三维畸形波生成、演化过程中异常大波无量纲波面时间过程

Fig. 7 Normalized surface elevations of abnormal large waves occurring during the generation and evolution process of the three-dimensional freak wave for Case 2

下载: 全尺寸图片幻灯片

图 8 工况3三维畸形波生成、演化过程中异常大波无量纲波面时间过程

Fig. 8 Normalized surface elevations of abnormal large waves occurring during the generation and evolution process of the three-dimensional freak wave for Case 3

下载: 全尺寸图片幻灯片

图 9 工况4三维畸形波生成、演化过程中异常大波无量纲波面时间过程

Fig. 9 Normalized surface elevations of abnormal large waves occurring during the generation and evolution process of the three-dimensional freak wave for Case 4

下载: 全尺寸图片幻灯片

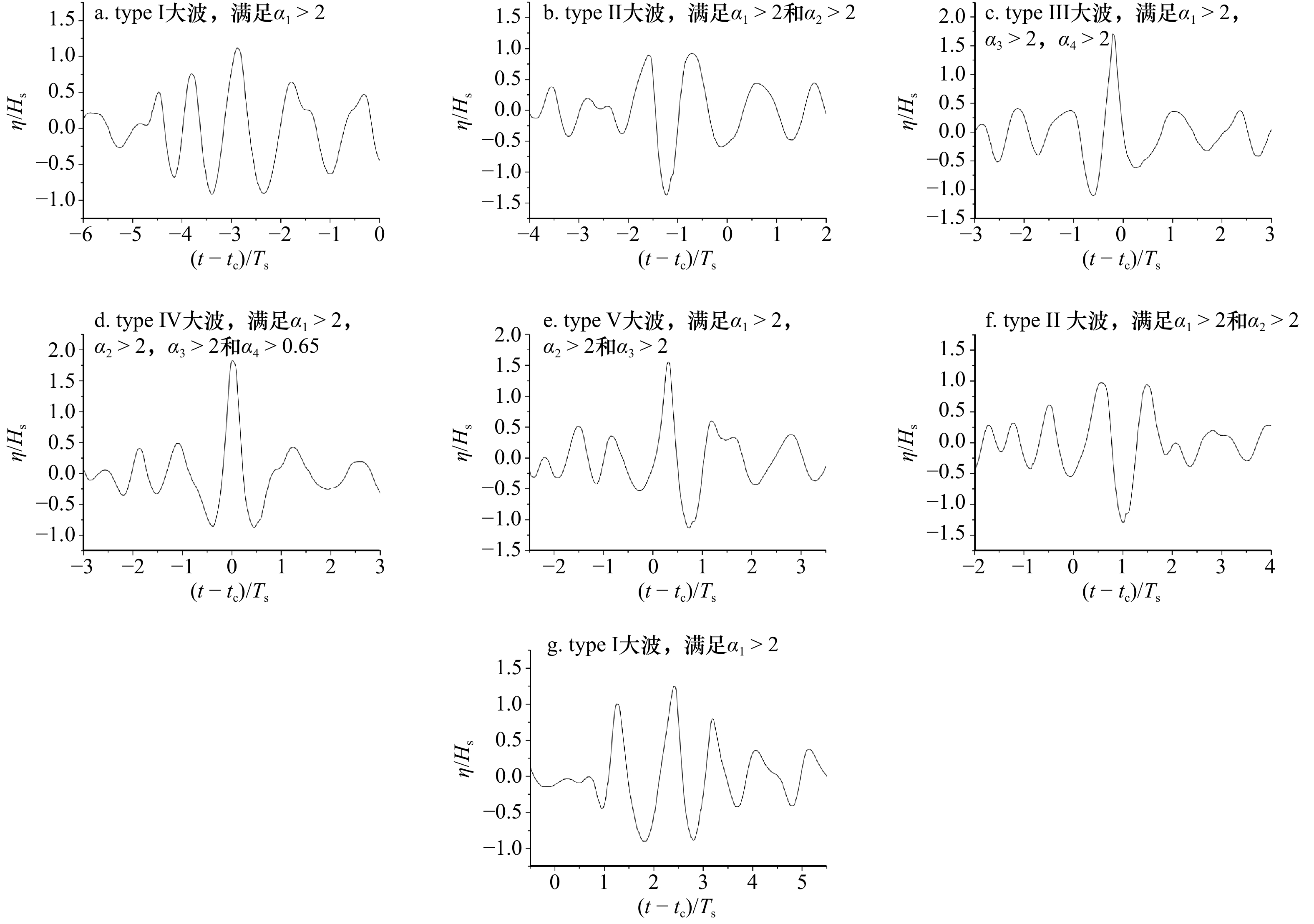
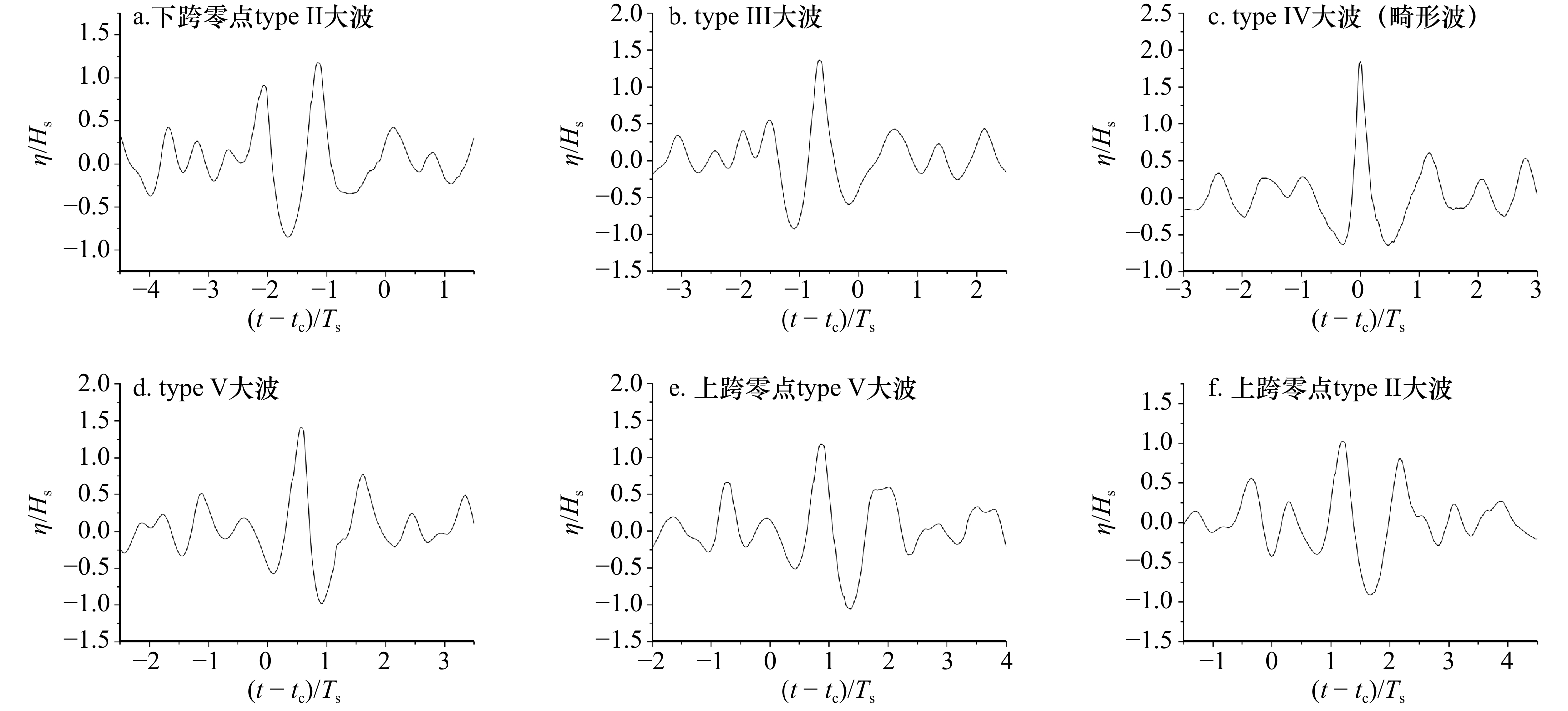
表 1 二维异常大波特征参数统计结果

Tab. 1 Freak wave parameters of abnormal large waves for the two-dimensional freak wave

大波类型	参数	图片编号
type I大波	α_1-up = 2.0, α_2-up= 1.2, α_3-up= 1.6, α_4-up= 0.55或α_1-down = 2.0, α_2-down= 1.4, α_3-down= 1.3, α_4-down = 0.55	图4a
type II大波	α_1-up = 2.3, α_2-up= 4.0, α_3-up= 1.5, α_4-up= 0.39或α_1-down = 2.3, α_2-down=1.8, α_3-down= 2.2, α_4-down = 0.40	图4b
type III大波	α_1-up = 2.3, α_2-up= 1.6, α_3-up= 3.4, α_4-up= 0.73或α_1-down = 2.8, α_2-down= 3.6, α_3-down= 2.9, α_4-down = 0.61	图4c
type IV大波	α_1-up = 2.7, α_2-up= 2.0, α_3-up= 4.0, α_4-up= 0.68或α_1-down = 2.7, α_2-down = 3.3, α_3-down = 2.1, α_4-down = 0.68	图4d
type V大波	α_1-up = 2.7, α_2-up = 3.1, α_3-up= 2.6, α_4-up= 0.58或α_1-down = 2.1, α_2-down= 2.7, α_3-down= 1.2, α_4-down = 0.75	图4e
type II大波	α_1-up = 2.3, α_2-up= 2.0, α_3-up = 1.7, α_4-up = 0.43或α_1-down = 2.2, α_2-down = 1.5, α_3-down = 3.8, α_4-down = 0.40	图4f
type I大波	α_1-up = 2.1, α_2-up= 1.1, α_3-up= 1.7, α_4-up= 0.58或α_1-down = 2.1, α_2-down= 1.5, α_3-down= 1.3, α_4-down = 0.58	图4g

下载: 导出CSV

表 2 三维异常大波的上跨零点特征参数

Tab. 2 Freak wave parameters of abnormal large waves for three-dimensional cases based on zero-up crossing analysis

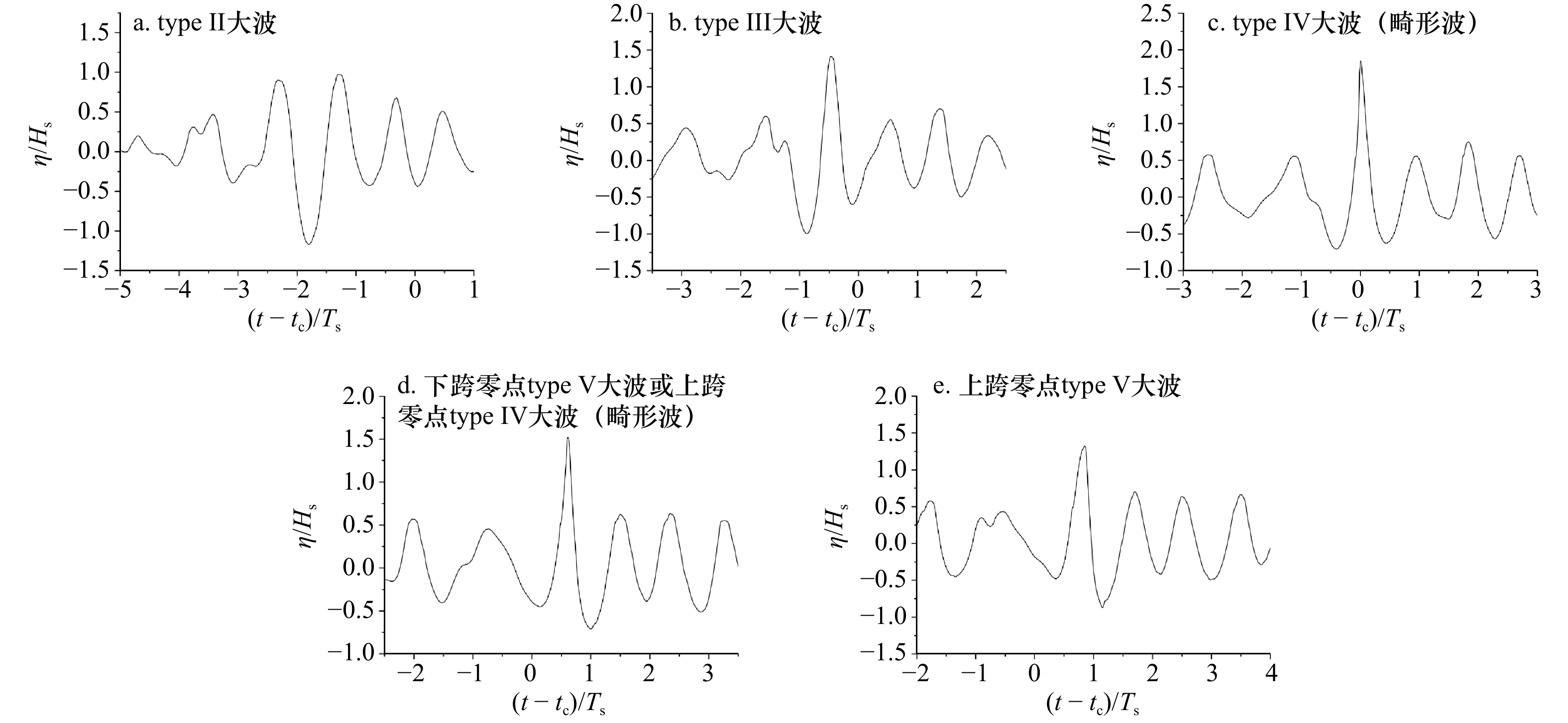
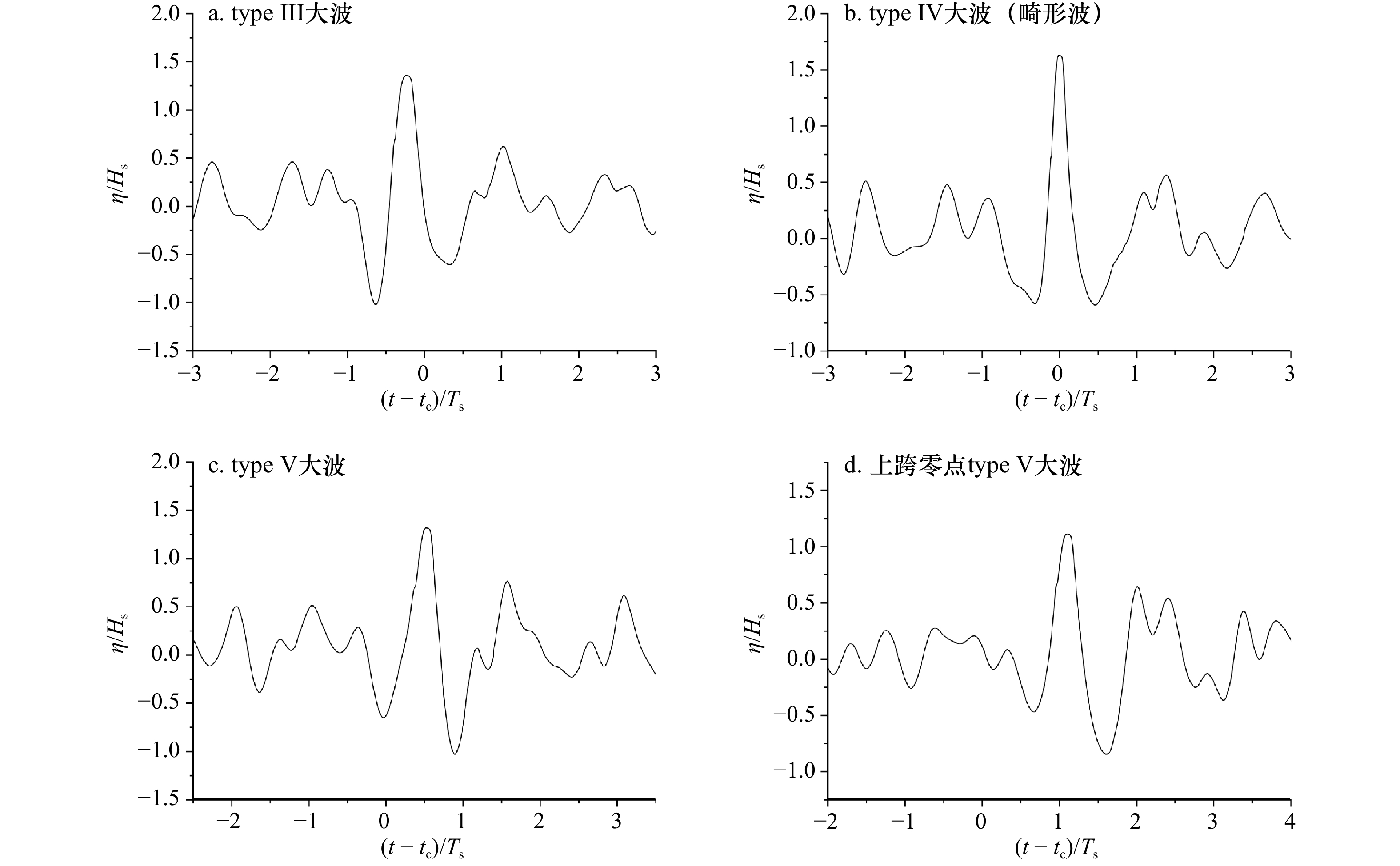
工况	阶段	类型	α_1-up	α_2-up	α_3-up	α_4-up	（t_m– t_c）/T_s	（x_m− x_c）/L_s
工况1	第2	II	2.1	2.4	1.4	0.44	−1.80	−1.30
	第3	III	2.0	1.3	2.2	0.70	−0.40	−0.50
	第4	IV	2.5	2.0	2.9	0.76	0.00	0.00
	第5	V	2.2	2.4	2.0	0.60	0.90	0.80
工况2	第3	III	2.0	1.3	2.9	0.69	−0.20	−0.30
	第4	IV	2.2	2.1	3.1	0.73	0.00	0.00
	第5	V	2.0	2.6	2.0	0.56	1.10	1.10
工况3	第3	III	2.0	1.3	3.3	0.70	−0.60	−0.60
	第4	IV	2.5	2.7	3.1	0.74	0.00	0.00
	第5	V	2.2	3.3	2.4	0.53	0.90	0.90
	第6	II	2.0	2.9	1.8	0.53	1.70	1.20
工况4	第3	III	2.0	1.3	3.0	0.71	−0.60	−0.70
	第4	IV	2.6	2.3	3.2	0.73	0.00	0.00
	第5	V	2.1	2.0	2.7	0.54	0.80	0.70
工况5	第3	III	2.0	1.7	2.6	0.7	−0.10	−0.10
	第4	IV	2.3	3.7	2.6	0.75	0.00	0.00
	第5	V	2.1	3.7	2.7	0.61	0.50	0.40
	第6	II	2.0	3.8	1.7	0.53	1.10	0.70
工况6	第3	III	2.0	1.7	3.9	0.65	−0.10	−0.10
	第4	IV	2.1	2.0	4.1	0.67	0.00	0.00
	第5	V	2.4	2.7	2.8	0.59	0.40	0.30
	第6	II	2.0	2.9	1.8	0.45	1.10	0.70

下载: 导出CSV

表 3 三维异常大波的下跨零点特征参数

Tab. 3 Freak wave parameters of abnormal large waves for three-dimensional cases based on zero-down crossing analysis

工况	阶段	类型	α_1-down	α_2-down	α_3-down	α_4-down	（t_m– t_c）/T_s	（x_m− x_c） /L_s
工况1	第2	II	2.1	1.7	2.0	0.45	−1.80	−1.30
	第3	III	2.4	2.8	2.1	0.59	−0.40	−0.50
	第4	IV	2.5	3.1	2.2	0.73	0.00	0.00
	第5	V	2.0	2.3	1.5	0.77	0.70	0.60
工况2	第3	III	2.4	3.4	2.0	0.57	−0.20	−0.30
	第4	IV	2.2	3.5	2.0	0.74	0.00	0.00
	第5	V	2.0	2.2	1.8	0.67	0.50	0.50
工况3	第2	II	2.0	1.8	2.6	0.58	−1.60	−1.20
	第3	III	2.3	3.5	2.3	0.60	−0.60	−0.60
	第4	IV	2.5	4.5	2.0	0.74	0.00	0.00
	第5	V	2.0	6.9	1.1	0.71	0.60	0.60
工况4	第3	III	2.1	2.3	2.1	0.51	−0.80	−0.90
	第4	IV	2.5	2.8	2.1	0.75	0.00	0.00
	第5	V	2.0	2.5	1.6	0.69	0.30	0.20
工况5	第3	III	2.2	5.5	2.0	0.57	−0.20	−0.20
	第4	IV	2.3	4.6	2.0	0.75	0.00	0.00
	第5	V	2.1	4.7	1.8	0.80	0.10	0.10
工况6	第3	III	2.0	3.0	2.0	0.59	−0.10	−0.10
	第4	IV	2.1	2.9	2.0	0.68	0.00	0.00
	第5	V	2.0	2.9	1.7	0.72	0.10	0.10

下载: 导出CSV

参考文献(21)

[1]	Gemmrich J, Thomson J. Observations of the shape and group dynamics of rogue waves[J]. Geophysical Research Letters, 2017, 44(4): 1823−1830.
[2]	崔成, 严冰, 左书华. 基于OpenFOAM三维多向畸形波数值模拟研究[J]. 海洋工程, 2019, 37(1): 46−55, 74. Cui Cheng, Yan Bing, Zuo Shuhua. Numerical simulation of three-dimensional multi-directional freak waves based on OpenFOAM[J]. The Ocean Engineering, 2019, 37(1): 46−55, 74.
[3]	Stansell P. Distributions of freak wave heights measured in the North Sea[J]. Applied Ocean Research, 2004, 26(1/2): 35−48.
[4]	Stansell P. Distributions of extreme wave, crest and trough heights measured in the North Sea[J]. Ocean Engineering, 2005, 32(8/9): 1015−1036.
[5]	Cui Cheng, Zhang Ningchuan, Pei Yuguo, et al. Numerical study on generation and evolution of freak waves[J]. Journal of Ship Mechanics, 2012, 16(12): 1373−1384.
[6]	Fochesato C, Grilli S, Dias F. Numerical modeling of extreme rogue waves generated by directional energy focusing[J]. Wave Motion, 2007, 44(5): 395−416. doi: 10.1016/j.wavemoti.2007.01.003
[7]	Barratt D, Bingham H B, Adcock T A A. Nonlinear evolution of a steep, focusing wave group in deep water simulated with OCEANWAVE3D[J]. Journal of Offshore Mechanics and Arctic Engineering, 2020, 142(2): 021201. doi: 10.1115/1.4044989
[8]	Waseda T, Kinoshita T, Tamura H. Evolution of a random directional wave and freak wave occurrence[J]. Journal of Physical Oceanography, 2009, 39(3): 621−639. doi: 10.1175/2008JPO4031.1
[9]	Mori N, Onorato M, Janssen P A E M. On the estimation of the kurtosis in directional sea states for freak wave forecasting[J]. Journal of Physical Oceanography, 2011, 41(8): 1484−1497. doi: 10.1175/2011JPO4542.1
[10]	Johannessen T B, Swan C. A laboratory study of the focusing of transient and directionally spread surface water waves[J]. Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences, 2001, 457(2008): 971−1006. doi: 10.1098/rspa.2000.0702
[11]	Barratt D, Bingham H B, Taylor P H, et al. Rapid spectral evolution of steep surface wave groups with directional spreading[J]. Journal of Fluid Mechanics, 2021, 907: A30. doi: 10.1017/jfm.2020.835
[12]	She K, Created C A, Easson W J. Experimental study of three-dimensional breaking wave kinematics[J]. Applied Ocean Research, 1997, 19(5/6): 329−343.
[13]	Pei Yuguo, Zhang Ningchuan, Zhang Yunqiu. Efficient generation of freak waves in laboratory[J]. China Ocean Engineering, 2007, 21(3): 515−523.
[14]	Zhao Xizeng, Hu Changhong, Sun Zhaochen. Numerical simulation of extreme wave generation using VOF method[J]. Journal of Hydrodynamics, 2010, 22(4): 466−477. doi: 10.1016/S1001-6058(09)60078-0
[15]	Cui Cheng, Zhang Ningchuan, Zuo Shuhua, et al. A study on kinematics characteristics of freak wave[J]. China Ocean Engineering, 2013, 27(3): 391−402. doi: 10.1007/s13344-013-0034-8
[16]	Tao Aifeng, Xie Shuya, Wu Di, et al. The effects on water particle velocity of wave peaks induced by nonlinearity under different time scales[J]. Journal of Marine Science and Engineering, 2021, 9(7): 748. doi: 10.3390/jmse9070748
[17]	Klinting P, Sand S E. Analysis of prototype freak waves[C]//Proceedings of Coastal Hydrodynamic, Newark: American Society of Civil Engineers. 1987: 618−632.
[18]	Kriebel D L, Alsina M V. Simulation of extreme waves in a background random sea[C]//Proceedings of 10th International Offshore and Polar Engineering Conference. Seattle, Washington, USA: ISOPE, 2000: 1−2.
[19]	Cui Cheng, Zhang Ningchuan, Kang Haigui, et al. An experimental and numerical study of the freak wave speed[J]. Acta Oceanologica Sinica, 2013, 32(5): 51−56. doi: 10.1007/s13131-013-0313-5
[20]	Magnusson A K, Trulsen K, Aarnes O J, et al. “Three sisters” measured as a triple rogue wave group[C]//Proceedings of the 38th International Conference on Ocean, Offshore and Arctic Engineering. Glasgow, UK: ASME, 2019: 96837.
[21]	付睿丽, 马玉祥, 董国海. 深水随机波列中畸形波统计特征的研究[J]. 海洋学报, 2021, 43(10): 81−89. Fu Ruili, Ma Yuxiang, Dong Guohai. Researches on statistical properties of freak waves in uni-directional random waves in deep water[J]. Haiyang Xuebao, 2021, 43(10): 81−89.