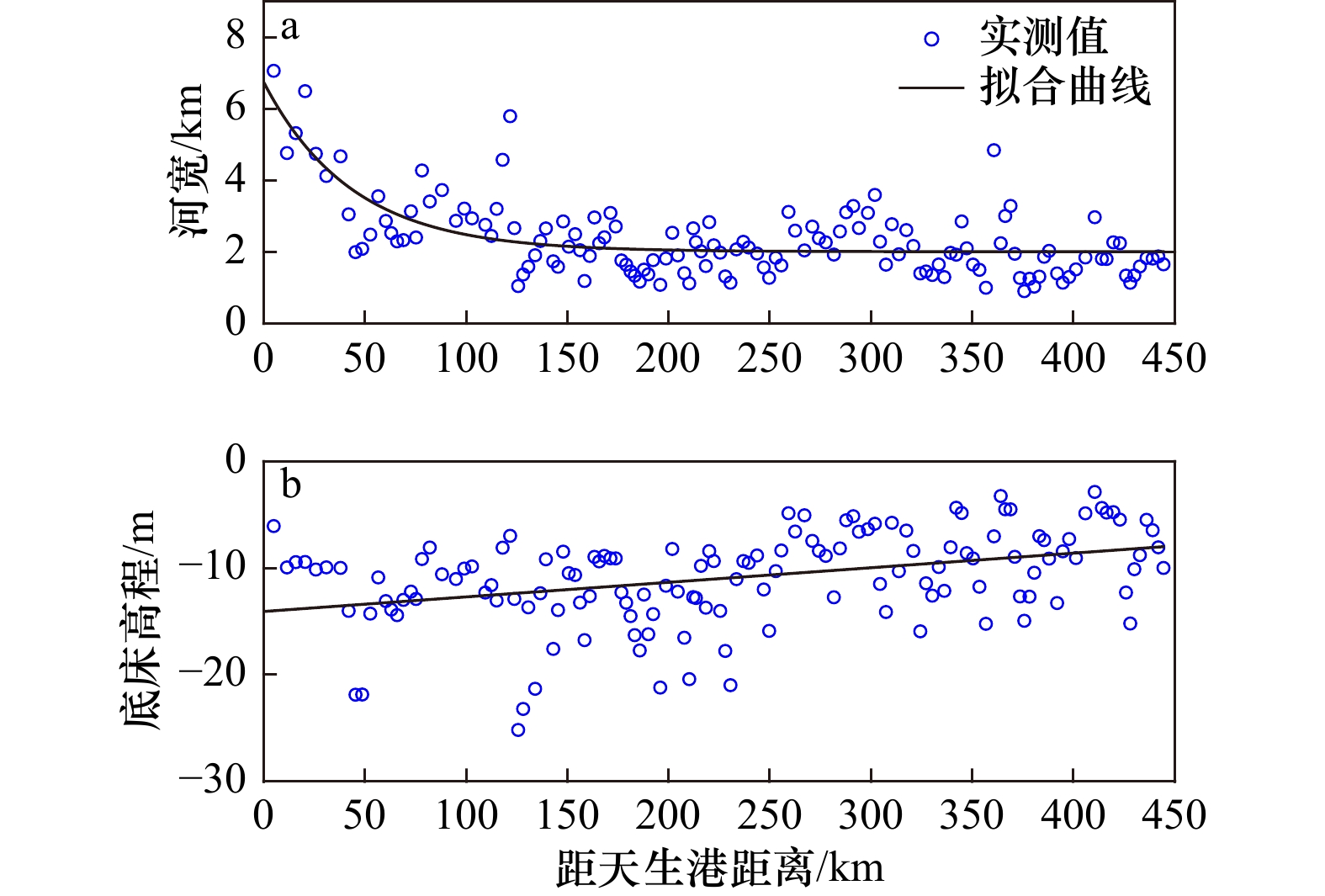
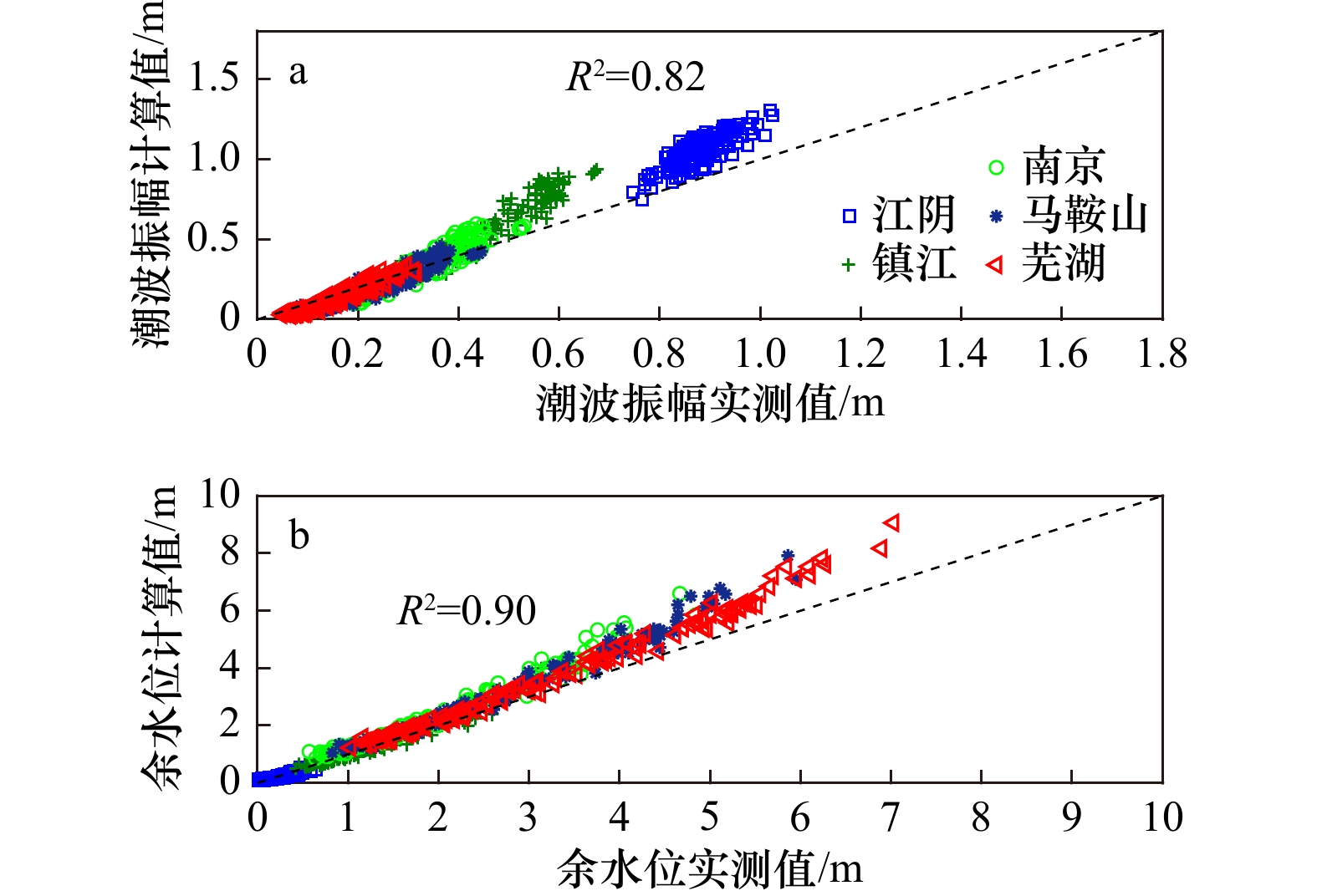
The evolution of estuarine backwater dynamics and its underlying mechanism: a case study of the Changjiang River Estuary
-
摘要: 河口回水区长度的时空演变对防洪、供水、航运等水资源高效开发利用具有重要指导意义。本文在经典河流回水理论的基础上考虑潮汐动力的影响，聚焦潮汐动力引起的回水效应问题，基于一维水动力解析模型，重新定义河口回水区上游界限（回水界），并以长江河口为例，探讨河口回水动力演变过程及其影响机制。结果表明：回水界距天生港的距离（即回水区长度）与上游流量、外海边界振幅分别具有显著的负相关和正相关关系，且基本为线性关系；回水界对径潮动力的响应比潮区界更为敏感，能有效表征河口感潮河段径潮动力格局演变；长江河口回水界位置具有明显的季节性差异，其春季和秋季回水界位于芜湖附近（春季和秋季分别距天生港419 km和367 km），冬季回水界位于感潮河段以上区域，夏季潮汐动力引起的回水效应基本可忽略；长江河口潮流因子和径潮相互作用因子控制的余水位梯度的季节性变化，是长江河口回水界位置变动的主导因素。Abstract: The spatio-temporal evolution of backwater influence length in estuaries is essential with regard to the sustainable water resources management in general, such as flood control, water supply, and shipping. In this study, based on the classical riverine backwater theory, we focus on the backwater effect caused by tidal dynamics, making use of a one-dimensional hydrodynamic analytical model to redefine the upstream boundary of the backwater zone (namely backwater limit) in estuaries. The model was subsequently applied to the Changjiang River Estuary to explore the evolution of estuarine backwater dynamics and its underlying mechanism. Results show that the distance from Tianshenggang to backwater limit (i.e., the backwater influence length) has a negatively linear correlation with the upstream river discharge and positively linear correlation with the tidal amplitude of seaward boundary, respectively. The response of the backwater limit to tidal river dynamics is more sensitive than that of the tidal limit, which can effectively characterize the evolution of the tidal river dynamics in tidal reach. The location of the backwater limit in the Changjiang River Estuary has apparent seasonal difference, being 419 km away from Tianshenggang during the spring and 367 km during autumn. The location is situated above the tidal reach during the winter, while the backwater effect caused by tidal dynamics during the summer is negligible. It was shown that the seasonal variations of residual water level slope induced by tide and tide-river interaction are the dominant factors for controlling the location of the backwater limit in the Changjiang River Estuary.
-
图 2 长江河口平均河宽（a）和底床高程（b）的沿程变化及其曲线拟合
Fig. 2 Longitudinal variations of the tidally averaged channel width (a) and bed elevation (b) along the Changjiang River Estuary and their fitting curves
图 3 实测潮波振幅（a）、余水位（b）与解析模型计算值的对比
Fig. 3 Comparison of the observed tidal amplitude (a) and residual water level (b) with analytically computed results
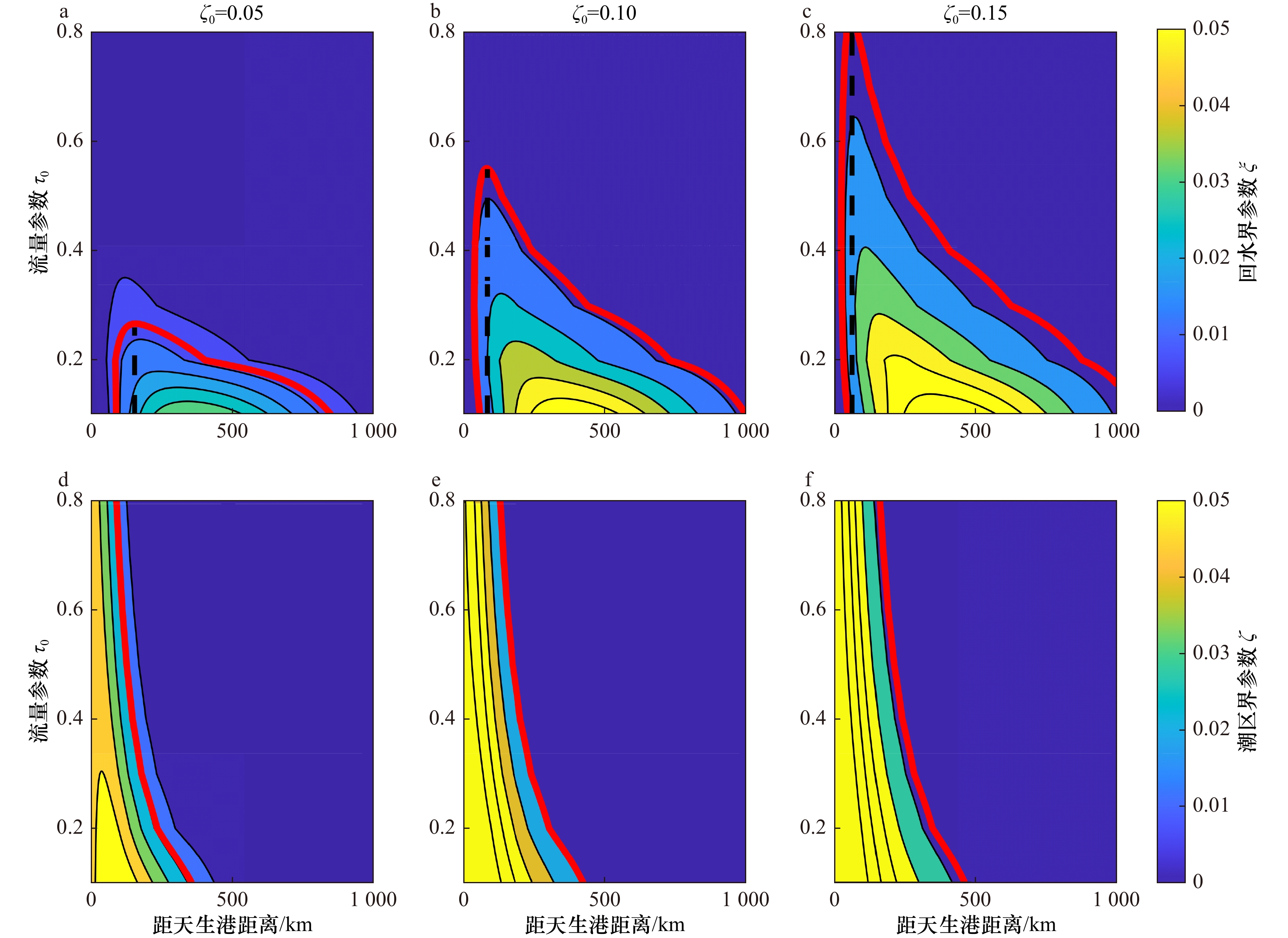
图 4 不同径潮动力组合条件下回水界参数ξ（a–c）和潮区界参数ζ（d–f）的沿程变化等值线图
a–c中红色实线对应回水界ξ = 0.01；d–f中红色实线对应潮区界ζ = 0.01
Fig. 4 Contour plots of longitudinal variations of parameters of backwater limit ξ (a–c) and tidal limit ζ (d–f) under different tide-river conditions
The thick red lines represent the locations of the backwater limit ξ = 0.01 in a–c and tidal limit ζ = 0.01 in d–f
图 5 不同径潮动力组合条件下回水界距天生港距离的等值线图
Fig. 5 Contour plot of distance from Tianshenggang to backwater limit under different tide-river conditions
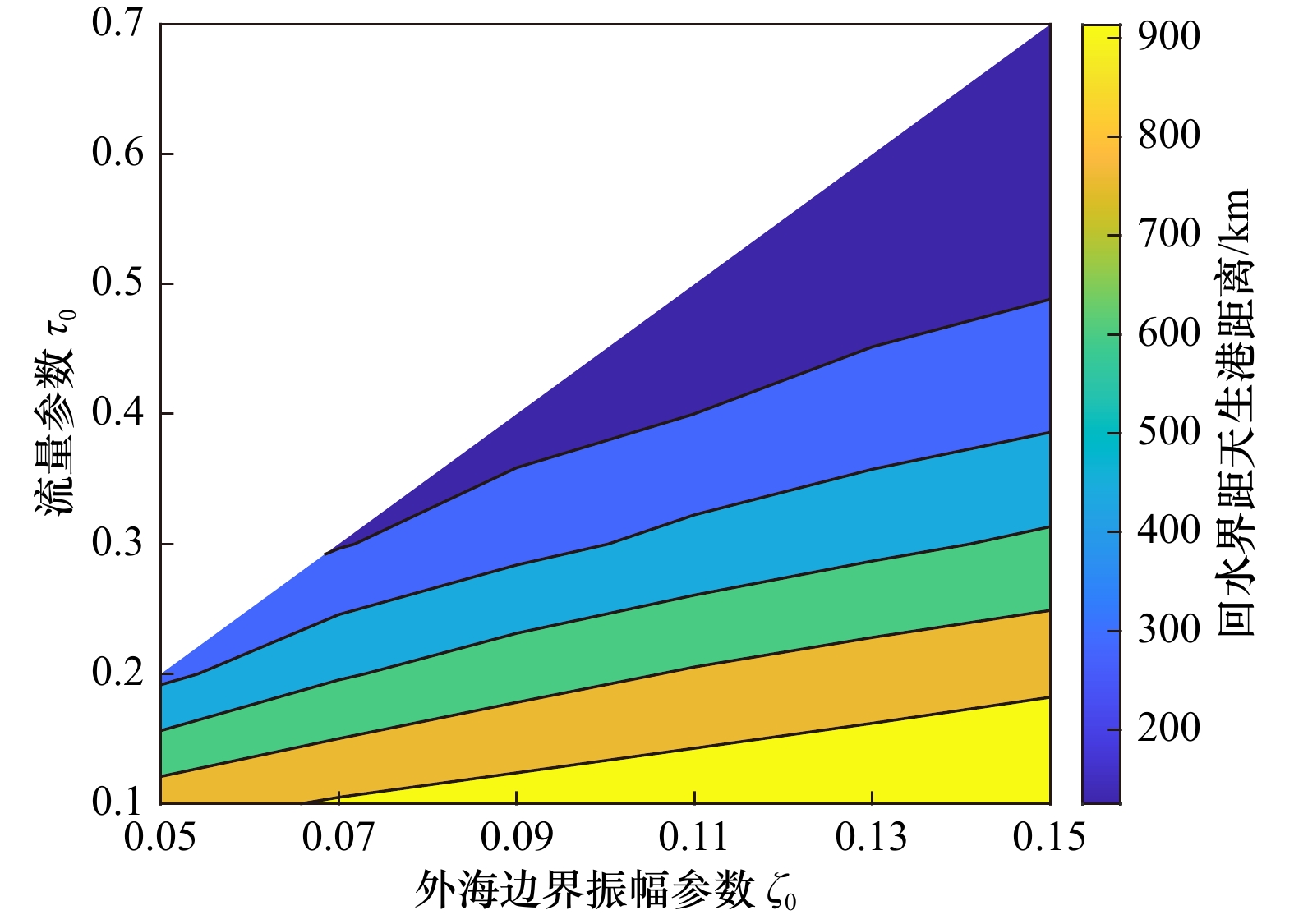
图 6 长江河口月均流量及外海边界振幅变化（a）及对应条件下回水界参数ξ的时空变化等值线（b）
b中红色实线对应回水界ξ = 0.01
Fig. 6 Variations of monthly averaged discharge and tidal amplitude of seaward boundary (a) and contour plot of spatial-temporal variation of the backwater limit ξ under the corresponding conditions (b) in the Changjiang River Estuary
The thick red lines represent the location of the backwater limit ξ = 0.01 in b
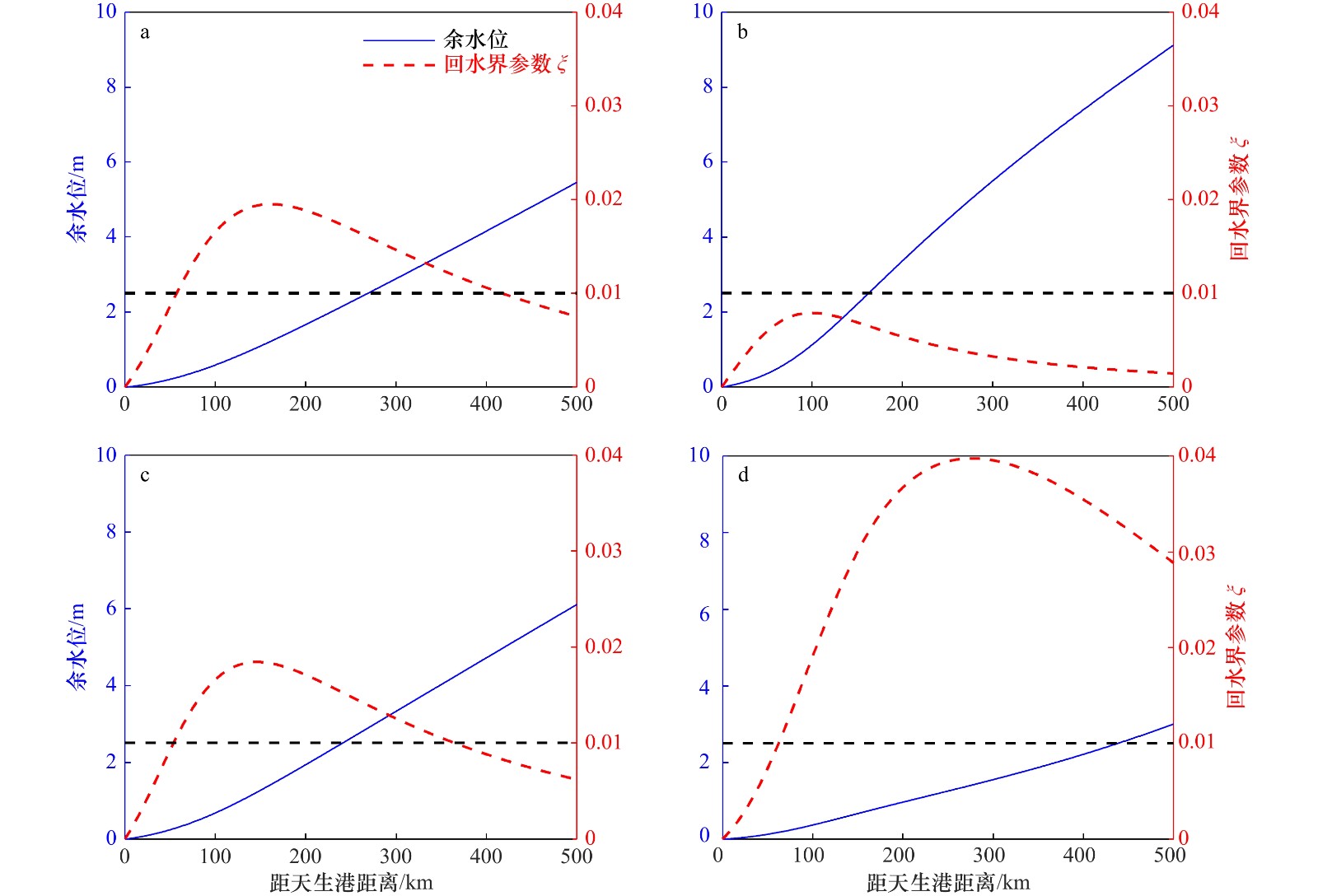
图 7 不同季节长江河口余水位及回水界参数ξ的沿程变化
a. 春季Q = 24 037 m3/s、$\eta _0 $ = 0.98 m；b. 夏季Q = 41 379 m3/s、$\eta _0 $ = 0.97 m；c. 秋季Q = 26 861 m3/s、$\eta _0 $ = 1.05 m；d. 冬季Q = 13 610 m3/s、$\eta _0 $ = 0.99 m；黑色虚线对应回水界参数ξ = 0.01
Fig. 7 Longitudinal variations of the residual water level and the parameter of backwater limit ξ in the Changjiang River Estuary in different seasons
a. Q = 24 037 m3/s and $\eta _0 $ = 0.98 m in spring; b. Q = 41 379 m3/s and $\eta _0 $ = 0.97 m in summer; c. Q = 26 861 m3/s and $\eta _0 $ = 1.05 m in autumn; d. Q = 13 610 m3/s and $\eta _0 $ = 0.99 m in winter. The dashed black lines correspond to the parameter of the backwater limit ξ = 0.01
图 8 不同季节长江河口余水位梯度分解项ft和ftr的沿程变化
a. 春季；b. 夏季；c. 秋季；d. 冬季
Fig. 8 Longitudinal variations of ft and ftr derived from the residual water level slope in the Changjiang River Estuary in different seasons
a. Spring; b. summer; c. autumn; d. winter
-
[1] Godin G, Martínez A. Numerical experiments to investigate the effects of quadratic friction on the propagation of tides in a channel[J]. Continental Shelf Research, 1994, 14(7/8): 723−748. [2] Zhang Min, Yang Hao, Tang Qibang, et al. Impacts of secondary and quarter-diurnal tidal species on backwater hydrodynamics in tidal rivers[J]. Advances in Water Resources, 2020, 143: 103660. doi: 10.1016/j.advwatres.2020.103660 [3] Guo Leicheng, Van Der Wegen M, Jay D A, et al. River-tide dynamics: exploration of nonstationary and nonlinear tidal behavior in the Yangtze River Estuary[J]. Journal of Geophysical Research: Oceans, 2015, 120(5): 3499−3521. doi: 10.1002/2014JC010491 [4] Dykstra S L, Dzwonkowski B. The propagation of fluvial flood waves through a backwater-estuarine environment[J]. Water Resources Research, 2020, 56(2): e2019WR025743. [5] Lamb M P, Nittrouer J A, Mohrig D, et al. Backwater and river plume controls on scour upstream of river mouths: implications for fluvio-deltaic morphodynamics[J]. Journal of Geophysical Research: Earth Surface, 2012, 117(F1): F01002. [6] Chow V T. Open Channel Hydraulics[M]. New York: McGraw-Hill, 1959. [7] Paola C, Mohrig D. Palaeohydraulics revisited: palaeoslope estimation in coarse-grained braided rivers[J]. Basin Research, 1996, 8(3): 243−254. doi: 10.1046/j.1365-2117.1996.00253.x [8] Swartz J M, Goudge T A, Mohrig D C. Quantifying coastal fluvial morphodynamics over the last 100 years on the lower Rio Grande, USA and Mexico[J]. Journal of Geophysical Research: Earth Surface, 2020, 125(6): e2019JF005443. [9] Brooke S A S, Ganti V, Chadwick A J, et al. Flood variability determines the location of lobe-scale avulsions on deltas: madagascar[J]. Geophysical Research Letters, 2020, 47(20): e2020GL088797. [10] Wu Chenliang, Nitterour J A. Impacts of backwater hydrodynamics on fluvial-deltaic stratigraphy[J]. Basin Research, 2020, 32(3): 567−584. doi: 10.1111/bre.12385 [11] Jones A E, Hardison A K, Hodges B R, et al. Defining a riverine tidal freshwater zone and its spatiotemporal dynamics[J]. Water Resources Research, 2020, 56(4): e2019WR026619. [12] Cai Huayang, Savenije H H G, Toffolon M. Linking the river to the estuary: influence of river discharge on tidal damping[J]. Hydrology and Earth System Sciences, 2014, 18(1): 287−304. doi: 10.5194/hess-18-287-2014 [13] Cai Huayang, Savenije H H G, Jiang C. Analytical approach for predicting fresh water discharge in an estuary based on tidal water level observations[J]. Hydrology and Earth System Sciences, 2014, 18(10): 4153−4168. doi: 10.5194/hess-18-4153-2014 [14] Cai Huayang, Savenije H H G, Jiang Chenjuan, et al. Analytical approach for determining the mean water level profile in an estuary with substantial fresh water discharge[J]. Hydrology and Earth System Sciences, 2016, 20(3): 1177−1195. doi: 10.5194/hess-20-1177-2016 [15] Cai Huayang, Savenije H H G, Garel E, et al. Seasonal behaviour of tidal damping and residual water level slope in the Yangtze River Estuary: identifying the critical position and river discharge for maximum tidal damping[J]. Hydrology and Earth System Sciences, 2019, 23(6): 2779−2794. doi: 10.5194/hess-23-2779-2019 [16] 张先毅, 黄竞争, 杨昊, 等. 长江河口潮波传播机制及阈值效应分析[J]. 海洋与湖沼, 2019, 50(4): 788−798. doi: 10.11693/hyhz20181200305Zhang Xianyi, Huang Jingzheng, Yang Hao, et al. The governing mechanism of tidal wave propagation and threshold effect in the Changjiang River Estuary[J]. Oceanologia et Limnologia Sinica, 2019, 50(4): 788−798. doi: 10.11693/hyhz20181200305 [17] 杨昊, 欧素英, 姚鹏, 等. 河口区斜压梯度对余水位的累积影响及其机制探讨[J]. 海洋学报, 2019, 41(1): 21−31.Yang Hao, Ou Suying, Yao Peng, et al. Cumulative effect of baroclinic gradient on the residual water level in estuaries and its underlying mechanism[J]. Haiyang Xuebao, 2019, 41(1): 21−31. [18] 蔡华阳, 杨昊, 郭晓娟, 等. 珠江磨刀门河口径潮动力耦合条件下余水位的多时空尺度分析[J]. 海洋学报, 2018, 40(7): 55−65.Cai Huayang, Yang Hao, Guo Xiaojuan, et al. Investigation of temporal-spatial distribution patterns of residual water level under the influence of tide-river interaction in the Modaomen Estuary, Zhujiang River[J]. Haiyang Xuebao, 2018, 40(7): 55−65. [19] 王文才, 李一平, 杜薇, 等. 长江感潮河段潮汐变化特征[J]. 水资源保护, 2017, 33(6): 121−124, 132. doi: 10.3880/j.issn.1004-6933.2017.06.19Wang Wencai, Li Yiping, Du Wei, et al. Tidal variation features of tidal reach of Changjiang River[J]. Water Resources Protection, 2017, 33(6): 121−124, 132. doi: 10.3880/j.issn.1004-6933.2017.06.19 [20] Zhang Eefeng, Savenije H H G, Chen Shenliang, et al. An analytical solution for tidal propagation in the Yangtze Estuary, China[J]. Hydrology and Earth System Sciences, 2012, 16(9): 3327−3339. doi: 10.5194/hess-16-3327-2012 [21] Savenije H H G, Toffolon M, Haas J, et al. Analytical description of tidal dynamics in convergent estuaries[J]. Journal of Geophysical Research: Oceans, 2008, 113(C10): C10025. doi: 10.1029/2007JC004408 [22] Van Rijn L C. Analytical and numerical analysis of tides and salinities in estuaries; Part I: tidal wave propagation in convergent estuaries[J]. Ocean Dynamics, 2011, 61(11): 1719−1741. doi: 10.1007/s10236-011-0453-0 [23] Dronkers J. Convergence of estuarine channels[J]. Continental Shelf Research, 2017, 144: 120−133. doi: 10.1016/j.csr.2017.06.012 [24] Savenije H H G. Salinity and Tides in Alluvial Estuaries[M]. Amsterdam: Elsevier Science, 2005. [25] 萨莫依洛夫 И В. 河口演变过程的理论及其研究方法[M]. 谢金赞, 译. 北京: 科学出版社, 1958.Samoylov И В. The Theory and Method of the Evolution Processes of the Estuaries[M]. Xie Jinzan, trans. Beijing: Science Press, 1958. [26] 杨云平, 李义天, 韩剑桥, 等. 长江口潮区和潮流界面变化及对工程响应[J]. 泥沙研究, 2012(6): 46−51. doi: 10.3969/j.issn.0468-155X.2012.06.007Yang Yunping, Li Yitian, Han Jianqiao, et al. Variation of tide limit and tidal current limit in Yangtze Estuary and its impact on projects[J]. Journal of Sediment Research, 2012(6): 46−51. doi: 10.3969/j.issn.0468-155X.2012.06.007 -
下载:
计量
- 文章访问数: 563
- HTML全文浏览量: 200
- PDF下载量: 66
- 被引次数: 0
