Medium and long term statistical prediction of sea surface height anomaly in the South China Sea based on evolutionary operator
-
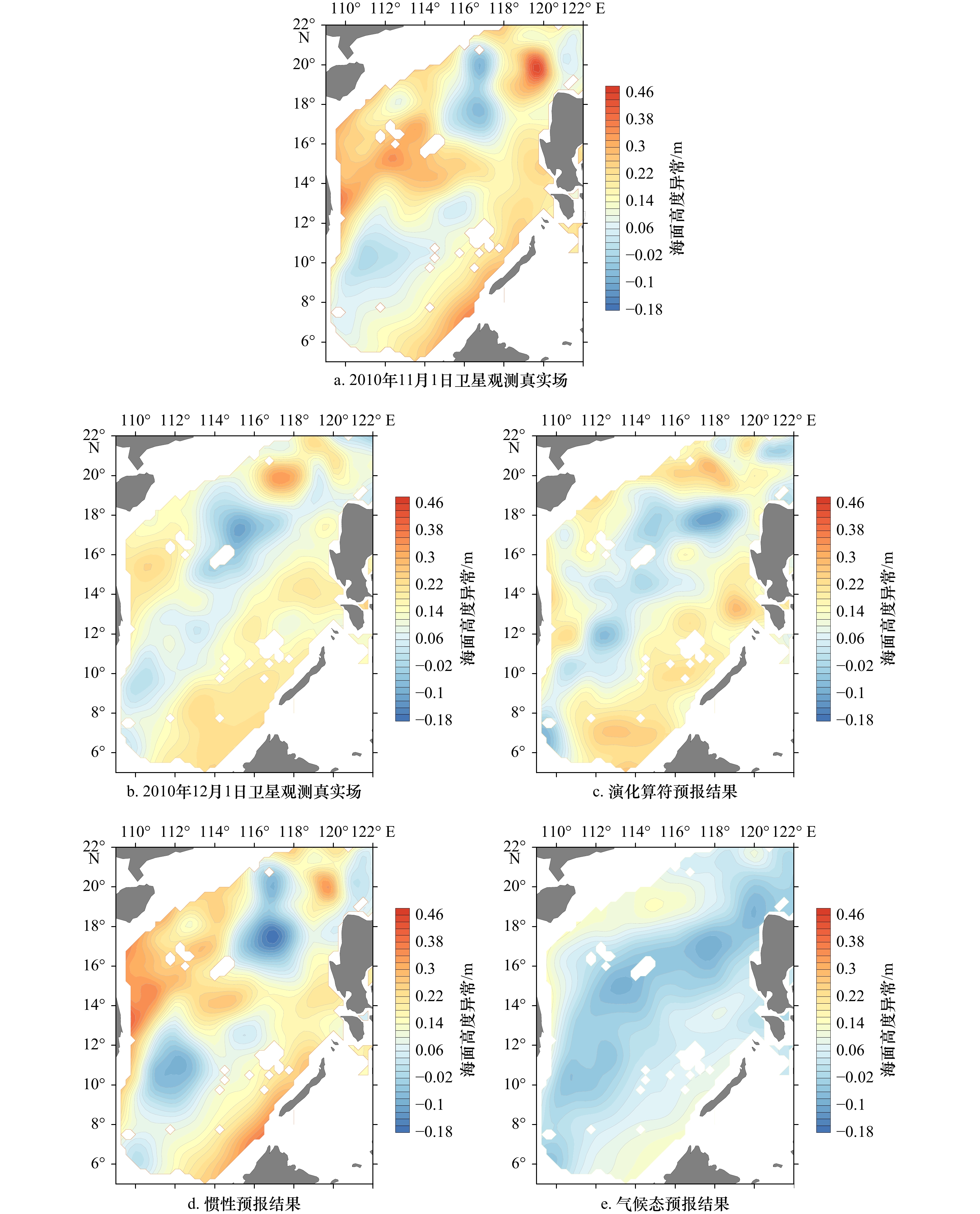
摘要: 水下移动平台行动时需要1～3个月左右海洋数值预测预报结果，但是当前数值预报技术受对应的气象驱动场预报时效的限制，难以提供10 d以上的数值预报产品。鉴于海水在动力热力上具有较大的惯性，海洋内区有其自身的演化规律，本研究设计了一种基于演化算符的统计预测方法，利用历史卫星遥感资料构建海洋状态变量中长期演化矩阵，并结合惯性预报模型，构建了最终的南海海洋中长期统计预报模型，能够提供1～60 d逐日的南海海面高度异常预测结果，开展数值试验验证了该方法的有效性，结果表明，在起报后15 d内，预报结果与卫星资料的相关系数均大于0.8，在起报60 d内，相关系数仍高于0.6。Abstract: When performing work, underwater platforms usually need about 1-month to 3-months ocean numerical forecasts results. However, the current numerical forecasting technology is limited to the corresponding weather-driven field forecasting, and it is difficult to provide numerical forecast products for more than 10 days. Considering that the seawater itself has large inertia physically, and the inner ocean area has its own evolutionary principles, a statistical prediction method based on evolutionary operator is proposed. The method uses historical satellite remote sensing data to construct a long-term evolution matrix of ocean state variables, and then combines with the inertial prediction model. The final medium- and long-term statistical prediction model of the South China Sea is constructed, which can provide the prediction results for 1 d to 60 d of daily sea surface height anomaly in the South China Sea. We carry out the numerical experiments to show the effectiveness of the method. The results show that the correlation coefficients between the forecast results and the satellite data are all higher than 0.8 within 15 days and higher than 0.6 within 60 days after the start date of forecast.
-
Key words:
- ocean forecast /
- evolution operator /
- medium- and long-range
-
1. 引言
温度、盐度和深度是影响海水声速的3大主要因素，其中温度对表层水面声速的影响尤为重要，并且表层海水声速受其影响变得较为复杂[1]。在高精度的多波束测深中，真实可靠的海底波束脚印坐标可借助高精度的声速剖面数据和精确的声线跟踪模型获得[2]，因此，声速剖面数据对波束脚印的精确计算起到决定性的作用。
海水声速测量的方法有两种，其一为直接测量法，利用声速剖面仪（Sound Velocity Profiler, SVP）通过声波在某一海水深度处固定距离上的传播时间或相位，直接获得声波传播速度的方法。通常又分为环鸣法、相位法、脉冲时间法、驻波干涉法及脉冲循环法等。其二为间接测量法，利用温盐深（Conductivity, Temperature, Depth, CTD）剖面仪测定每层海水的温度、盐度以及深度（静压力），再根据海水声速与温度、盐度、压力（深度）之间的经验公式，获取声速剖面[3]。在直接测量法中，虽然可借助高精度的SVP获取准确度较高的声速剖面数据，但是在实际水深测量过程中，也常因为表层温盐深变化的复杂性而没有布设足够密度的实测站点而导致代表性误差，从而给海底水深测量带入显著性误差[4]。在间接测量法中，国内外众多学者对一些声速经验公式进行了比对，分析了它们的适用范围和相关精度[5-7]，但是甚少对温盐深变化引起的声速误差做出定量化计算，也没有进一步对温盐深变化导致的海底波束脚印坐标误差做出定量化分析，尽管有些文献已经阐述了表层声速误差对波束脚印坐标计算的影响[8-9]。
为此，本文将先对温盐深变化引起的声速误差做定量化分析，然后根据声线跟踪模型和声速剖面计算声速变化对海底波束脚印坐标值的影响程度。此分析结果可为温盐深误差对多波束测深精度的评估工作提供借鉴作用。
2. 温盐深变化对声速的影响
近半个世纪以来，海洋科学家相继提出很多不同海水的声速经验公式，不同的公式有其不同的适用范围，尽管一些公式适用范围相同，但是公式形式差别较大，在不同的海域，由不同声速经验公式获得的声速差异较大，即使在同一海域，它们计算出来的声速同实际声速也存在着较大差异。目前对声速经验公式研究较多的是DelGrosso、Wilson和Chen-Miller 3种算法，相关的研究表明，DelGrosso的声速计算算法比Chen-Miller算法好[10]，Wilson公式也叫Wilson精确公式，虽然提出年代早，但直至现在还被公认为较为稳定的公式[6]。为此，本文研究使用DelGrosso和Wilson的声速计算公式做相关分析。
1974年，DelGrosso给出了一种计算海水声速的经验公式（简称“DelGrosso公式”）[11]，由此可推导出其声速误差模型公式，即：
$$ {\rm{d}}{C_{STP}}{\text{ = }}{\rm{d}}{C_T} + {\rm{d}}{C_S} + {\rm{d}}{C_P} +{\rm{ d}}{C_{STP}} \text{，} $$ (1) $$ \begin{split} {\rm{d}}{C_T} = & 0. 501\;109\;398\;873 \times 10{\rm{d}}T - 0. 550\;946\;843\;172 \\ &\times 10^{ - 1} \times [2T{\rm{d}}T + {({\rm{d}}T)^2}] + 0. 221\;535\;969\;240 \\ &\times10^{ - 3} \times [3{T^2}{\rm{d}}T + 3T{({\rm{d}}T)^2} + ({\rm{d}}T)^3] \text{，} \end{split} $$ (2) $$ \begin{split} {\rm{d}}{C_S}=& 0. 132\;952\;290\;781 \times 10^1 \times {\rm{d}}S \\ & +0. 128\;955\;756\;844 \times 10^{ - 3} \times [2S{\rm{d}}S + {({\rm{d}}S)^2}] \text{，} \end{split} $$ (3) $$ \begin{split} {\rm{ d}}{C_P}=& 0. 156\;059\;257\;041 \times {\rm{d}}P +0. 244\;998\;688\;441 \\ & \times 10^{ - 4} \times [2P{\rm{d}}P + {({\rm{d}}P)^2}] -0. 883\;392\;332\;513 \\ & \times10^{ - 8}[3{P^2}{\rm{d}}P + 3P{({\rm{d}}P)^2} + {({\rm{d}}P)^3}] \text{，} \end{split} $$ (4) $$ \begin{split} {\rm{d}}C_{STP} = &- 0. 127\;562\;783\;426 \times 10^{ - 1}(S{\rm{d}}T + T{\rm{d}}S + {\rm{d}}S{\rm{d}}T) \\ & +0. 635\;191\;613\;389 \times 10^{ - 2}(P{\rm{d}}T + T{\rm{d}}P + {\rm{d}}P{\rm{d}}T) \\ &+0. 265\;484\;716\;608 \times 10^{ - 7}[2T{P^2}{\rm{d}}T + {P^2}{({\rm{d}}T)^2} \\ &+2{T^2}P{\rm{d}}P + 4TP{\rm{d}}T{\rm{d}}P + 2P{\rm{d}}P{({\rm{d}}T)^2} + {T^2}{({\rm{d}}P)^2} \\ &+ 2T{\rm{d}}T{({\rm{d}}P)^2} + {({\rm{d}}T)^2}{({\rm{d}}P)^2}] - 0. 159\;349\;479\;045 \\ &\times10^{ - 5}[{P^2}{\rm{d}}T + 2TP{\rm{d}}P + 2P{\rm{d}}T{\rm{d}}P + T{({\rm{d}}P)^2} \\ &+ {\rm{d}}T({\rm{d}}P)^2 ]+ 0.522\;116\;437\;235 \times 10^{ - 9}\times [3T{P^2}{\rm{d}}P \\ & + 3TP{({\rm{d}}P)^2} +T{({\rm{d}}P)^3} + {P^3}{\rm{d}}T + 3{P^2}{\rm{d}}T{\rm{d}}P \\ & + 3P{\rm{d}}T{({\rm{d}}P)^2}+ {\rm{d}}T{({\rm{d}}P)^3}] -0. 438\;031\;096\;213 \\ & \times 10^{ - 6}[3{T^2}P{\rm{d}}T + 3TP{({\rm{d}}T)^2} +P{({\rm{d}}T)^3} + {T^3}{\rm{d}}P \\ & + 3{T^2}{\rm{d}}T{\rm{d}}P + 3T{({\rm{d}}T)^2}{\rm{d}}P+ P{({\rm{d}}T)^3}] \\ & - 0. 161\;674\;495\;909 \times 10^{ - 8}[2S{P^2}{\rm{d}}S + {P^2}{({\rm{d}}S)^2} \\ & +2S^2P{\rm{d}}P+4SP{\rm{d}}S{\rm{d}}P+2P{\rm{d}}P{({\rm{d}}S)}^2+S^2{({\rm{d}}P)}^2 \\& +2S{\rm{d}}S{({\rm{d}}P)^2} + {({\rm{d}}S)^2}{({\rm{d}}P)^2}] + 0. 968\;403\;156\;410 \\ &\times 10^{ - 4} \times [2TS{\rm{d}}T + S{({\rm{d}}T)^2} + {T^2}{\rm{d}}S + 2T{\rm{d}}T{\rm{d}}S \\ & + {\rm{d}}S{({\rm{d}}T)^2}] + 0. 485\;639\;620\;015\times 10^{ - 5}[P{S^2}{\rm{d}}T \\&+ T{S^2}{\rm{d}}P + {S^2}{\rm{d}}T{\rm{d}}P + 2TPS{\rm{d}}S + 2SP{\rm{d}}T{\rm{d}}S \\ &+ 2ST{\rm{d}}P{\rm{d}}S + 2S{\rm{d}}T{\rm{d}}P{\rm{d}}S + TP({\rm{d}}S)^2 + P{\rm{d}}T{({\rm{d}}S)^2} \\ &+ T{\rm{d}}P{({\rm{d}}S)^2} + {\rm{d}}T{\rm{d}}P{({\rm{d}}S)^2}]- 0. 340\;597\;039\;004 \\ & \times 10^{ - 3}[PS{\rm{d}}T + TP{\rm{d}}S + P{\rm{d}}T{\rm{d}}S + TS{\rm{d}}P \\& + S{\rm{d}}T{\rm{d}}P+ T{\rm{d}}S{\rm{d}}P + {\rm{d}}T{\rm{d}}S{\rm{d}}P] \text{，} \end{split} $$ (5) 式中，dCSTP为总声速误差，dCT为温度引起的声速误差，dCS为盐度引起的声速误差，dCP为压力引起的声速误差，dCSTP为温盐压引起的声速误差，单位均为m/s；T为温度，dT为温度误差，单位为℃；S为盐度，dS为盐度误差；P为压强，dP为压强误差，单位为kg/cm2。
1960年，Wilson给出了一种计算海水声速的经验公式（简称“Wilson公式”）[12]，由此可推导出其声速误差模型公式，即：
$$ {\rm{d}}V{\text{ = }}{\rm{d}}{V_T}{\text{ + }}{\rm{d}}{V_P} + {\rm{d}}{V_S} + {\rm{d}}{V_{STP}} \text{，} $$ (6) $$ \begin{split} {\rm{ d}}{V_T} = & 4.623\,3{\rm{d}}T -5.458\,5\times 10^{ - 2}[2T{\rm{d}}T + {({\rm{d}}T)^2}] \\ &+2.822 \times {10^{ - 4}}[3{T^2}{\rm{d}}T + 3T{({\rm{d}}T)^2} + {({\rm{d}}T)^3}] \\ &-5.07 \times {10^{ - 7}}[4{T^3}{\rm{d}}T + 6{T^2}{({\rm{d}}T)^2} \\ &+4T{({\rm{d}}T)^3} + {({\rm{d}}T)^4}] \text{，} \end{split} $$ (7) $$ \begin{split} {\rm{ d}}{V_P}=& 1.605\,18 \times 10^{-1} {\rm{d}}P+ 1.027\,9 \times 10^{- 5}[2P{\rm{d}}P+({\rm{d}}P)^2] \\ &+3.451\times 10^{-9}[3{P^2}{\rm{d}}P + 3P{({\rm{d}}P)^2} + {({\rm{d}}P)^3}] -3.503 \\ &\times 10^{ - 12}[4{P^3}{\rm{d}}P + 6{P^2}{({\rm{d}}P)^2} + 4P{({\rm{d}}P)^3} + {({\rm{d}}P)^4}] \text{，} \end{split} $$ (8) $$ {\rm{d}}{V_S}= 1.391{\rm{d}}S - 7.8 \times 10^{ - 2}[2S{\rm{d}}S - 70{\rm{d}}S + {({\rm{d}}S)^2}] \text{，} $$ (9) $$ \begin{split} {\rm{d}}{V_{STP}}=&(S-35)[-1.197 \times 10^{ - 2}{\rm{d}}T+ 2.61 \times 10^{ - 4}{\rm{d}}P \\ & - 1.96 \times 10^{ - 7} \times (2P{\rm{d}}P + {({\rm{d}}P)^2}) -2.09\times 10^{ - 6}\\ &\times(T{\rm{d}}P + P{\rm{d}}T + {\rm{d}}P{\rm{d}}T)]+ {\rm{d}}S[-1.197 \times 10^{ - 2} \\ &\times(T + {\rm{d}}T) +2.61 \times 10^{ - 4}(P + {\rm{d}}P) - 1.96 \\&\times 10^{ - 7}{(P + {\rm{d}}P)^2}) - 2.09 \times 10^{ - 6}(PT + T{\rm{d}}P \\ &+ P{\rm{d}}T + {\rm{d}}P{\rm{d}}T)] +P[ - 2.796 \times {10^{ - 4}}{\rm{d}}T \\ &+ 1.330\;2 \times {10^{ - 5}}(2T{\rm{d}}T + {({\rm{d}}T)^2})- 6.644 \\ &\times {10^{ - 8}}(3{T^2}{\rm{d}}T + 3T{({\rm{d}}T)^2}+ {({\rm{d}}T)^3})] \\&+ {\rm{d}}P[ - 2.796 \times {10^{ - 4}}(T+{\rm{d}}T) + 1.330\;2 \\ &\times {10^{ - 5}}{(T + {\rm{d}}T)^2} -6.644 \times {10^{ - 8}}{(T + {\rm{d}}T)^3}] \\ &+{P^2}[ - 2.391 \times {10^{ - 7}}{\rm{d}}T + 9.286 \times{10^{ - 10}} \\&\times (2T{\rm{d}}T + {({\rm{d}}T)^2})] + [2P{\rm{d}}P + {({\rm{d}}P)^2}] \\ &\times[ - 2.391 \times {10^{ - 7}}(T + {\rm{d}}T) + 9.286 \times {10^{ - 10}} \\ &\times{(T + {\rm{d}}T)^2}] - 1.745 \times{10^{ - 10}}\{{P^3}{\rm{d}}T + [3{P^2}{\rm{d}}P \\&+ 3P{({\rm{d}}P)^2} + {({\rm{d}}P)^3}](T + {\rm{d}}T)\} \text{，} \end{split}$$ (10) 式中，dV为总声速误差，dVT为温度引起的声速误差，dVS为盐度引起的声速误差，dVP为压力引起的声速误差，dVSTP为温盐压引起的声速误差，单位均为m/s；T为温度，dT为温度误差，单位为℃；S为盐度，dS为盐度误差；P为压强，dP为压强误差，单位为kg/cm2。
3. 声速变化对波束脚印坐标的影响
海水中声速与海水温度、盐度和静压力相关，并随着它们的变化而变化[13]，由于很难获得声速随深度变化的函数，通常只能借助声速剖面仪获得一定深度间隔的声速剖面，并根据声速变化，借助Snell法则，沿声线跟踪，获得波束海底投射点在船体坐标系下的坐标[14-15]。在每个水层中，由于只知道该层上界和下界声速，因此通常假设声速在该层以常梯度g传播，采用类似处理方法处理其他各水层，并沿着声线跟踪到海底，即实现常梯度声线跟踪和波束海底坐标计算[16]。
假设声线从换能器发射，经过N个水层，每个水层上界zi声速为Ci，下界zi+1声速为Ci+1，层内声速以常梯度gi变化，则i层内声速函数为
$$ {C_i}(z) = {C_i} + {g_i}(z - {z_i}) , $$ (11) 而声速常梯度gi的计算式为
$$ {g_i} = ({C_{i + 1}} - {C_i})/({z_{i + 1}} - {z_i}), $$ (12) 由式（12）推导出声速常梯度gi误差公式为
$$ {\rm{d}}{g_i} = ({\rm{d}}{C_{i + 1}} - {\rm{d}}{C_i})/({z_{i + 1}} - {z_i}). $$ (13) 如图1所示，在常梯度gi声速变化下，声线在第i层内的传播轨迹为一连续的、带有一定曲率半径为Ri的弧段[17]，若Snell常数为p，则Ri为
$$ {R_i} = - 1/p{g_i} . $$ (14) 假设第i层内声线传播的Harmonic平均声速为
$ {C_{{H_i}}} $ ，则声线在此层内传播的水平位移Δyi和时间ti为[18]$$ \begin{split} \Delta {y_i} &= {R_i}(\sin {\alpha _i} - \sin {\alpha _{i + 1}}) = (\cos{\theta _{i + 1}} - \cos{\theta _i})/p{g_i}\\ &=[(\cos{\theta _{i + 1}} - \cos{\theta _i})/\sin {\theta _i}]{C_i}/{g_i} \\ &=\left\{\sqrt {1 - {{[p({C_i} + {g_i}\Delta {z_i})]}^2}} - \sqrt {1 - {{(p{C_{i + 1}})}^2}} \right\}/p{g_i} \end{split} , $$ (15) 用dC和dgi代入式（15），得出水平位移Δyi的误差公式为
$$ \begin{split} {\rm{d}}(\Delta {y_i}) =& \left\{\sqrt {1 - {{\left\{p[({C_i} + {\rm{d}}{C_i}) + ({g_i} + {\rm{d}}{g_i})\Delta {z_i}]\right\} }^2}} \right.\\ &-\left.\sqrt {1-{{[p({C_{i + 1}} +{\rm{ d}}{C_{i + 1}})]}^2}} \right\} /p({g_i} + {\rm{d}}{g_i}) \\ &-\left\{\sqrt {1 - {{[p({C_i} + {g_i}\Delta {z_i})]}^2}} - \sqrt {1 - {{(p{C_{i + 1}})}^2}} \right\} /p{g_i}, \end{split} $$ (16) 声线在每个水柱层的旅行时间ti为
$$ \begin{split} {t_i} = &\frac{{{R_i}({\alpha _i} - {\alpha _{i + 1}})}}{{{C_{{H_i}}}}} = [\arccos (p{C_i} +p{g_i}\Delta {z_i}) \\ & -\arccos (p{C_i})]\times \ln (1 + {g_i}\Delta {z_i}/{C_i})/p{g_i}^2\Delta {z_i} , \end{split}$$ (17) $ {C_{{H_i}}} $ 为第i层内声线传播的Harmonic平均声速。同理，用dC和dgi代入式（17），得出每层声线旅行时间误差公式为
$$ \begin{split} {\rm{d}}{t_i} = &\Biggr\{ \ln \Biggr[1 + \frac{{({g_i} + {\rm{d}}{g_i})\Delta {z_i}}}{{{C_i} + {\rm{d}}{C_i}}}\Biggr]\arccos \{p[{C_i} + {\rm{d}}{C_i} + ({g_i}\\ & + {\rm{d}}{g_i})\Delta {z_i}]\} -\arccos [p({C_i} + {\rm{d}}{C_i})]\Biggr\} /p\Delta {z_i}{({g_i} + {\rm{d}}{g_i})^2} \\ &-\Biggr\{ \ln \left[1 + \frac{{{g_i}\Delta {z_i}}}{{{C_i}}}\right]\arccos [p({C_i} + {g_i}\Delta {z_i})] \\ &-\arccos (p{C_i})\Biggr\} /p{g_i}^2\Delta {z_i} . \end{split} $$ (18) 在分层声线跟踪时，除了计算整层的垂直位移、水平位移和传播时间外，还需要依据传播剩余时间计算剩余层的垂直位移和水平位移。假设声线在第i层内传播时，声线在该层内r点处（图1）结束，此时剩余时间tr等于波束单程旅行时间tall减去第i层以前累计的传播时间，则声线在剩余层内的垂直位移Δzr和水平位移Δyr为
$$ \Delta {y_r} = {R_i}(\sin {\alpha _i} - \sin {\alpha _r}) , $$ (19) $$ \Delta {z_r} = {R_i}\cos {\alpha _r} - {R_i}\cos {\alpha _i} = {t_r}{C_{{H_r}}} , $$ (20) 而这里的
$ {C_{{H_r}}} $ 取整个水柱的平均声速CH，其计算公式为$$ {C_H} = (z - {z_0}){\left[\sum\limits_{i = 1}^N {\frac{1}{{{g_i}}}} {\rm{ln}}\left(1 + \frac{{{g_i}\Delta {z_i}}}{{{C_i}}}\right)\right]^{ - 1}} . $$ (21) 由式（20）和（21）可得出最后一个水层的垂直深度误差d（Δzr）为
$$ \begin{split} {\rm{d}}(\Delta {z_r}) =& {t_r}\left\{(z - {z_0}){\left[\sum\limits_{i = 1}^N {\frac{1}{{{g_i} + {\rm{d}}{g_i}}}} {\rm{ln}}\left(1 + \frac{{({g_i} + {\rm{d}}{g_i})\Delta {z_i}}}{{{C_i} + {\rm{d}}{C_i}}}\right)\right]^{ - 1}} \right.\\ & -\left.(z - {z_0}){\left[\sum\limits_{i = 1}^N {\frac{1}{{{g_i}}}} {\rm{ln}}\left(1 + \frac{{{g_i}\Delta {z_i}}}{{{C_i}}}\right)\right]^{ - 1}}\right\} , \end{split} $$ (22) 则声线传播总的垂直位移z和水平位移y为
$$ y = \sum\limits_{i = 1}^{N - 1} {\Delta {y_i}} + \Delta {y_r} , $$ (23) $$ z = \sum\limits_{i = 1}^{N - 1} {\Delta {z_i}} + \Delta {z_r} . $$ (24) 4. 结果与分析
在本次的实验中，采用加拿大RBR XR-420温盐深仪在海南万宁某海域采集相关的温度、盐度和压力数据进行分析。XR-420 温盐深仪温度的测量范围为−5～35℃，精度为±0.002℃；电导的测量范围为0～70 mS/cm，精度为±0.003 mS/cm；深度测量范围为10～6 600 m，精度为满量程的0.05%。在该海域布设了4站测量站点（图2），每个站点间隔1 000 m左右，形成一个类似正方形的区域。采集的温盐压数据一共有3列，分别为电导率、温度和压强，它们的单位分别mS/cm、Degrees_C、deciBars，需要将它们转换成DelGrosso和Wilson的声速经验公式的单位，即ppt、℃、kg/cm2。实验中的多波束声呐数据，为Kongsberg公司的Simrad EM3002多波束回声测深仪所采集，声呐工作频率为300 kHz，扇区最大开角为130°，最大探测波束为254个，斜距分辨率为5 cm，深度分辨率为1 cm。以下分别分析温度、盐度和深度的变化对声速的影响，以及它们的变化对多波束海底波束脚印坐标的影响。
如图3所示，采用Wilson、DelGrosso声速经验公式计算4个站点的声速曲线分别用不同线段表示，由图可知，Wilson声速经验公式计算的4个站点的声速差值比DelGrosso声速经验公式计算的差值大。从纵深方向看，Wilson声速经验公式计算值是先变大后变小，DelGrosso声速经验公式计算值是随着深度增加逐渐变小。在水深25 m以浅的水域，两种声速经验公式在同一站点的计算值差值较大，而在25～60 m的水域，两者的计算值差值较小。
图 3 两种声速经验公式计算的4个站点的声速剖面Figure 3. The sound velocity profiles of four stations calculated by two empirical sound velocity formulas在盐度和深度两个参量不变的情况下，温度增加1℃后，分别采用两种声速经验公式计算4个站点声速的差值，如图4所示。温度增加1℃后计算出来的声速均比之前有所增大，声速增值在2.1～2.8 m/s之间，呈现出声速差值随水深增大而增大的趋势，但是两种声速经验公式计算同一站点的声速差值比较接近。
图 4 温度变化1℃后两种声速经验公式计算同一站点的声速差值Figure 4. The sound velocity difference after the 1℃ change of temperature calculated by two empirical sound velocity formulas at the same station在温度和深度两个参量不变的情况下，盐度增加1后，分别采用两种声速经验公式计算4个站点声速的差值，如图5所示。Wilson声速经验公式计算的4个站点盐度变化前后的声速差值呈现随水深增大而变小的规律；而DelGrosso声速经验公式计算的声速差值呈现随水深增大而变大的规律。并且，两者计算同一站点的声速差值相差太大。
图 5 盐度变化1后两种声速经验公式计算同一站点的声速差值Figure 5. The sound velocity difference after the 1 change of salinity calculated by two empirical sound velocity formulas at the same station在温度和盐度两个参量不变的情况下，深度增加10 m后，分别采用两种声速经验公式计算4个站点声速的差值，如图6所示。Wilson声速经验公式计算的4个站点深度变化前后的声速差值呈现随水深增大而变化较小的规律；而DelGrosso声速经验公式计算的声速差值呈现随水深增大而变小的规律。并且，两者计算同一站点的声速差值在25 m以浅水域相差太大，而在25～60 m水域声速差值比较接近。
图 6 深度变化10 m后两种声速经验公式计算同一站点的声速差值Figure 6. The sound velocity difference after the 10 m change of depth calculated by two empirical sound velocity formulas at the same station分别在温度变化1℃、盐度变化1、深度变化10 m的情况下，统计了4个站点两种声速经验公式计算的声速变化均值，如表1所示。其中当盐度变化1时，两种声速公式计算的变化均值相差较大，差值约为0.3 m/s；而当温度变化1℃和深度变化10 m时，两种声速公式计算的变化均值相差较小，差值在0.01 m/s以内。
表 1 4个站点2种声速经验公式计算的声速变化均值Table 1. The mean value of sound velocity variation calculated by two empirical sound velocity formulas at four stations参量变化值 Wilson公式计算值/（m·s–1） DelGrosso公式计算值/（m·s–1） 温度变化1℃ 2.582 2.572 盐度变化1 1.408 1.102 深度变化10 m 0.160 0.162 在分析了温度、盐度及深度变化对声速的影响之后，需要进一步分析3个参量的变化对多波束海底波束脚印坐标的影响。根据图3的声速剖面曲线，认为DelGrosso声速经验公式计算4个站点的声速较Wilson公式稳定，故采用DelGrosso公式计算随3个参量变化的声速变化值。
在分析温度、盐度及深度变化对波束脚印坐标影响规律的实验中，选择一个多波束条带第2001 Ping至2020 Ping 中左舷入射角60°～5°（外边缘波束至中央波束方向）的多波束数据进行声线跟踪获得相应的波束脚印坐标。将3个参量变化引起的声速变化值添加至变化前的声速剖面数据中，进而根据声线跟踪模型计算3个参数变化前后引起的波束脚印坐标值变化量。当温度变化1℃时，波束脚印3个轴坐标变化均呈现出随入射角增大而减小的趋势，如图7所示，这个现象可由式（15）解释，波束水平位移量与入射角是一种递减函数关系，而波束方位角由波束分配角和姿态角确定，是一个定量，因此，波束在X和Y轴的坐标值变化量与入射角也是一种递减函数关系；当盐度变化1时，波束脚印3个轴坐标变化也呈现出同样的变化趋势，如图8所示；而当深度变化10 m时，波束脚印3个轴坐标变化呈现出一种无序的现象，即无法判断3个坐标值变化随入射角变化的规律，如图9所示。
图 7 温度变化1°C后引起的坐标值变化量Figure 7. The coordinate value change caused by the 1 °C change of temperature
图 8 盐度变化1后引起的坐标值变化量Figure 8. The coordinate value change caused by the 1 change of salinity
图 9 深度变化10 m后引起的坐标值变化量Figure 9. The coordinate value change caused by the 10 m change of depth为了更好地揭示温盐深变化对波束脚印坐标值的影响规律，将20 Ping多波束数据中每个入射角的变化值取平均值绘制变化曲线图，如图10所示，当温度增加1℃或者盐度增加1后，波束脚印3个轴坐标值变化趋势为随着入射角增大而减小，X轴和Y轴坐标值变化率一致，而Z轴变化率较大；而当深度增加10 m后，波束脚印3个轴坐标值随入射角的变化率几乎处于水平状态，X轴和Y轴坐标值变化率也一致，而Z轴变化率较大。
图 10 温盐深变化引起的各个入射角波束坐标变化均值Figure 10. The mean value of beam coordinate variation of each incident angle caused by the variation of temperature, salinity and depth5. 结论
本文主要讨论了温度、盐度和深度三者的变化对海水声速的影响，进而对多波束海底波束脚印坐标的影响。针对研究海域的水文特点，分别采用了Wilson和DelGrosso 两种声速经验公式计算了温度、盐度及深度的变化值所引起的海水声速变化量。然后，在原始声速剖面文件中加入三者所引起的声速变化量，最后通过声线跟踪模型计算海底波束脚印坐标的变化值。实验结果表明，温度变化1℃所引起的海水声速变化较盐度变化1、深度变化10 m所引起的声速变化量大，其中深度变化所引起海水声速变化量最小。在影响波束脚印坐标方面，3个参量的变化引起的Z轴坐标值变化量均大于X、Y轴坐标值的变化量，而且Z轴坐标值变化量最高可达深度的0.6%左右。其中，温度和盐度的变化引起的3轴坐标值变化量随入射角的增大而减小，而深度变化引起的3轴坐标值变化量几乎不随入射角的变化而变化。本文的研究结果揭示了温度、盐度、深度的测量误差所引起的波束脚印坐标误差不可忽略，尤其在高精度的深海测量中，温度和盐度误差可以给水深测量结果带入较大的误差。为此，只有借助高精度的温盐深仪和遵守相关测量规范，充分重视温盐深误差给多波束测深带来的重要影响，尽可能地削弱温盐深误差给多波束测深带入的误差，才能获得满意的高精度测量成果。
-
图 1 3种预报方法与真实值的相关系数
Fig. 1 Correlation coefficients between three forecasting methods and real values
图 2 3种预报方法与真实值的均方根误差
Fig. 2 Root mean square error between three forecasting methods and real values
图 3 海面高度异常统计预报结果与卫星观测结果
Fig. 3 Statistical prediction results and satellite observation results of sea surface height anomalies
图 4 海面高度异常预测结果与卫星观测结果相对于预测起始点的增量
Fig. 4 The increment of prediction results and satellite observation results relative to the predicted starting point of sea surface height anomaly
图 5 演化算符预报与惯性预报方差的曲线拟合
Fig. 5 Curve fitting of evolutionary operator prediction and inertial prediction error
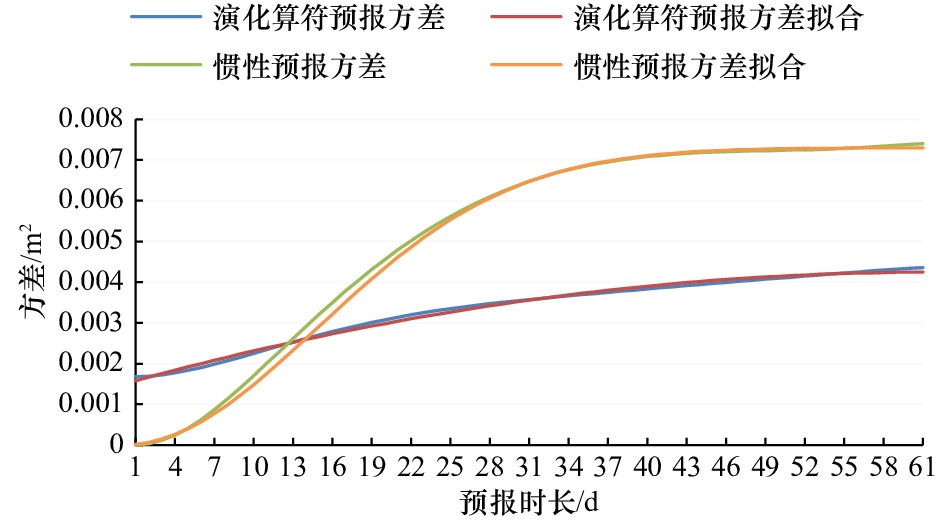
图 6 综合模型与3种模型的均方根误差与相关系数对比
a. 4种预报方法与真实值的均方根误差；b. 4种预报方法与真实值的相关系数
Fig. 6 Comparison of root mean square error and correlation coefficients between the comprehensive model and the three models
a. Four forecasting methods with real values root mean square error; b. correlation coefficients between four forecasting methods and real values
-
[1] 乔方利. 海洋动力环境要素数值预报关键技术[J]. 海洋开发与管理, 2006, 23(5): 59−60. doi: 10.3969/j.issn.1005-9857.2006.05.017Qiao Fangli. Key technology of NWP: Dynamic elements of marine environment[J]. Ocean Development and Management, 2006, 23(5): 59−60. doi: 10.3969/j.issn.1005-9857.2006.05.017 [2] 徐洋, 齐久成, 李清, 等. 美国海军业务化海洋预报系统综述[J]. 舰船科学技术, 2016, 38(5): 142−146. doi: 10.3404/j.issn.1672-7619.2016.05.030Xu Yang, Qi Jiucheng, Li Qing, et al. Overview of US navy operational ocean prediction system[J]. Ship Science and Technology, 2016, 38(5): 142−146. doi: 10.3404/j.issn.1672-7619.2016.05.030 [3] Carman J C, Eleuterio D P, Gallaudet T C, et al. The national earth system prediction capability: Coordinating the giant[J]. Bulletin of the American Meteorological Society, 2016, 98(2): 239−252. [4] 杨德全, 郝日栩, 何健新, 等. 统计预报方法在海洋预报中的应用研究进展[J]. 海洋信息, 2019, 34(2): 1−9.Yang Dequan, Hao Rixu, He Jianxin, et al. Research progress of statistical forecasting methods in ocean prediction[J]. Marine Information, 2019, 34(2): 1−9. [5] Barnston A G, Smith T M. Specification and prediction of global surface temperature and precipitation from global SST using CCA[J]. Journal of Climate, 1996, 9(11): 2660−2697. doi: 10.1175/1520-0442(1996)009<2660:SAPOGS>2.0.CO;2 [6] Landman W A, Mason S J. Forecasts of near-global sea surface temperatures using canonical correlation analysis[J]. Journal of Climate, 2001, 14(18): 3819−3833. doi: 10.1175/1520-0442(2001)014<3819:FONGSS>2.0.CO;2 [7] Niedzielski T, Kosek W. Multivariate stochastic prediction of the global mean sea level anomalies based on TOPEX/Poseidon satellite altimetry[J]. Artificial Satellites, 2005, 40(3): 185−198. [8] Ubilava D, Helmers C G. Forecasting ENSO with a smooth transition autoregressive model[J]. Environmental Modelling & Software, 2013, 40: 181−190. [9] Garcia-Gorriz E, Garcia-Sanchez J. Prediction of sea surface temperatures in the western Mediterranean Sea by neural networks using satellite observations[J]. Geophysical Research Letters, 2007, 34(11): L11603. doi: 10.1029/2007GL029888 [10] 孙文, 王庆宾, 周睿, 等. 海平面异常序列预报方法的比较与分析[J]. 测绘通报, 2014(1): 46−49.Sun Wen, Wang Qingbin, Zhou Rui, et al. A comparison and analysis of methods of sea level anomaly time series forecasting[J]. Bulletin of Surveying and Mapping, 2014(1): 46−49. [11] 李晓静. 基于概率神经网络与多重分形的海温预测模型[J]. 统计与决策, 2017(18): 84−87.Li Xiaojing. Prediction model of sea surface temperature based on probabilistic neural network and multifractal[J]. Statistics and Decision, 2017(18): 84−87. [12] 陈亚飞, 王晓春, 刘屹岷. 中国近海海面高度异常资料再处理[J]. 海洋科学, 2016, 40(7): 151−159. doi: 10.11759/hykx20150818001Chen Yafei, Wang Xiaochun, Liu Yimin. Sea level anomaly reprocessing for Chinese coastal region[J]. Marine Sciences, 2016, 40(7): 151−159. doi: 10.11759/hykx20150818001 [13] Vybíral J. A variant of Schur's product theorem and its applications[J]. Advances in Mathematics, 2020, 368: 107140. doi: 10.1016/j.aim.2020.107140 [14] 邹颖俊, 王晓春, 何贤强. 基于统计学方法的HYCOM海洋预报结果评价[J]. 湘潭大学自然科学学报, 2018, 40(3): 104−108.Zou Yingjun, Wang Xiaochun, He Xianqiang. Evaluation of HYCOM ocean forecasting fields based on statistical method[J]. Natural Science Journal of Xiangtan University, 2018, 40(3): 104−108. [15] 梁广建, 苏晓亮, 李淑君, 等. 南海海域MODAS有效性检验及误差分析[J]. 海洋技术, 2013, 32(3): 40−45.Liang Guangjian, Su Xiaoliang, Li Shujun, et al. The validity examination and error analysis of MODAS in South China Sea[J]. Ocean Technology, 2013, 32(3): 40−45. [16] 张晓芳, 贾思洋, 张曙伟, 等. 海洋垂直剖面水温实时监测浮标系统研制与应用[J]. 海洋科学, 2016, 40(5): 109−114. doi: 10.11759/hykx20140507002Zhang Xiaofang, Jia Siyang, Zhang Shuwei, et al. Research and application of real-time monitoring buoy system for marine water temperatures of vertical profiles[J]. Marine Sciences, 2016, 40(5): 109−114. doi: 10.11759/hykx20140507002 -
下载:
下载:
计量
- 文章访问数: 206
- HTML全文浏览量: 136
- PDF下载量: 33
- 被引次数: 0
