波浪在局部可渗透水平海床上传播的解析解

倪云林; 滕斌

doi:10.12284/hyxb2021131

波浪在局部可渗透水平海床上传播的解析解

doi: 10.12284/hyxb2021131

倪云林^{1, 2,},
滕斌^1, ,

1.
大连理工大学海岸和近海工程国家重点实验室，辽宁大连 116024
2.
浙江海洋大学海洋工程装备学院，浙江舟山 316022

基金项目: 浙江省自然科学基金青年基金（LQ18E090006）

详细信息

作者简介:
倪云林（1986-），男，浙江省舟山市人，博士，主要从事缓坡方程研究。E-mail：oceannyl@zjou.edu.cn

通讯作者:
滕斌(1958-)，男，山东省日照市人，教授，主要从事波浪对海上建筑物作用研究。E-mail: bteng@dlut.edu.cn

中图分类号: P731.22
计量
- 文章访问数: 279
- HTML全文浏览量: 81
- PDF下载量: 17
- 被引次数: 0
出版历程
- 收稿日期: 2020-05-19
- 修回日期: 2021-01-21
- 网络出版日期: 2021-07-05
- 刊出日期: 2021-10-30

Analytical solution for waves propagating over a local permeable seabed

Ni Yunlin^{1, 2
,},
Teng Bin^{1
, ,}

1.
State Key Laboratory of Coastal and Offshore Engineering, Dalian University of Technology, Dalian 116024, China
2.
School of Marine Engineering Equipment, Zhejiang Ocean University, Zhoushan 316022, China

摘要

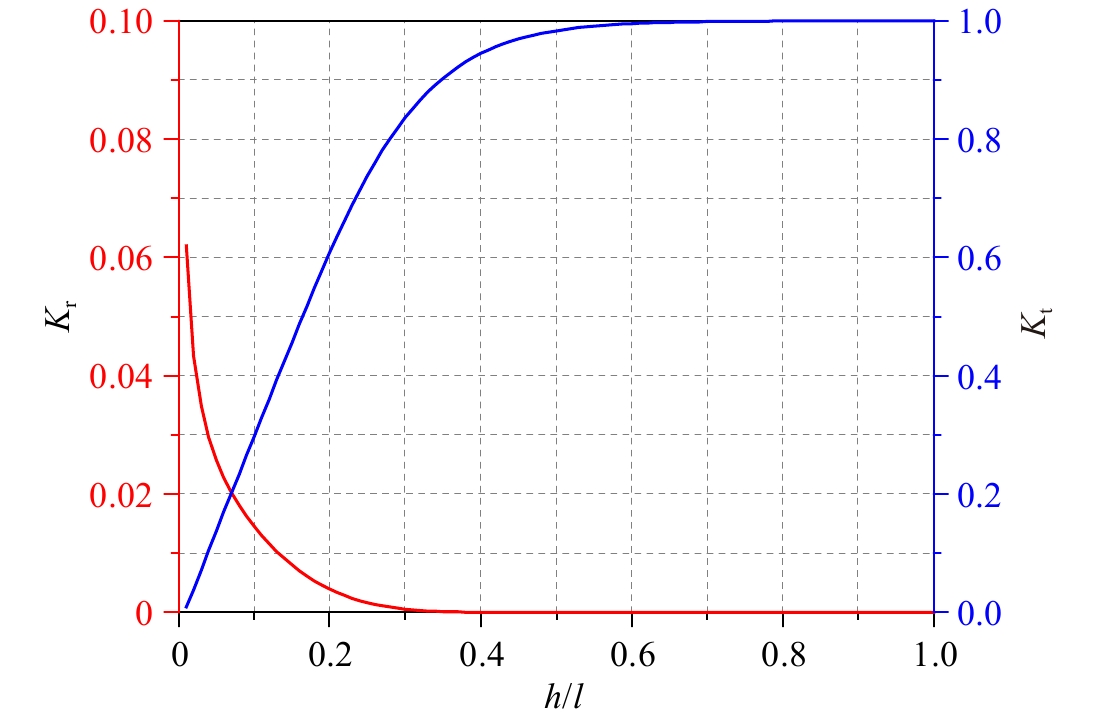
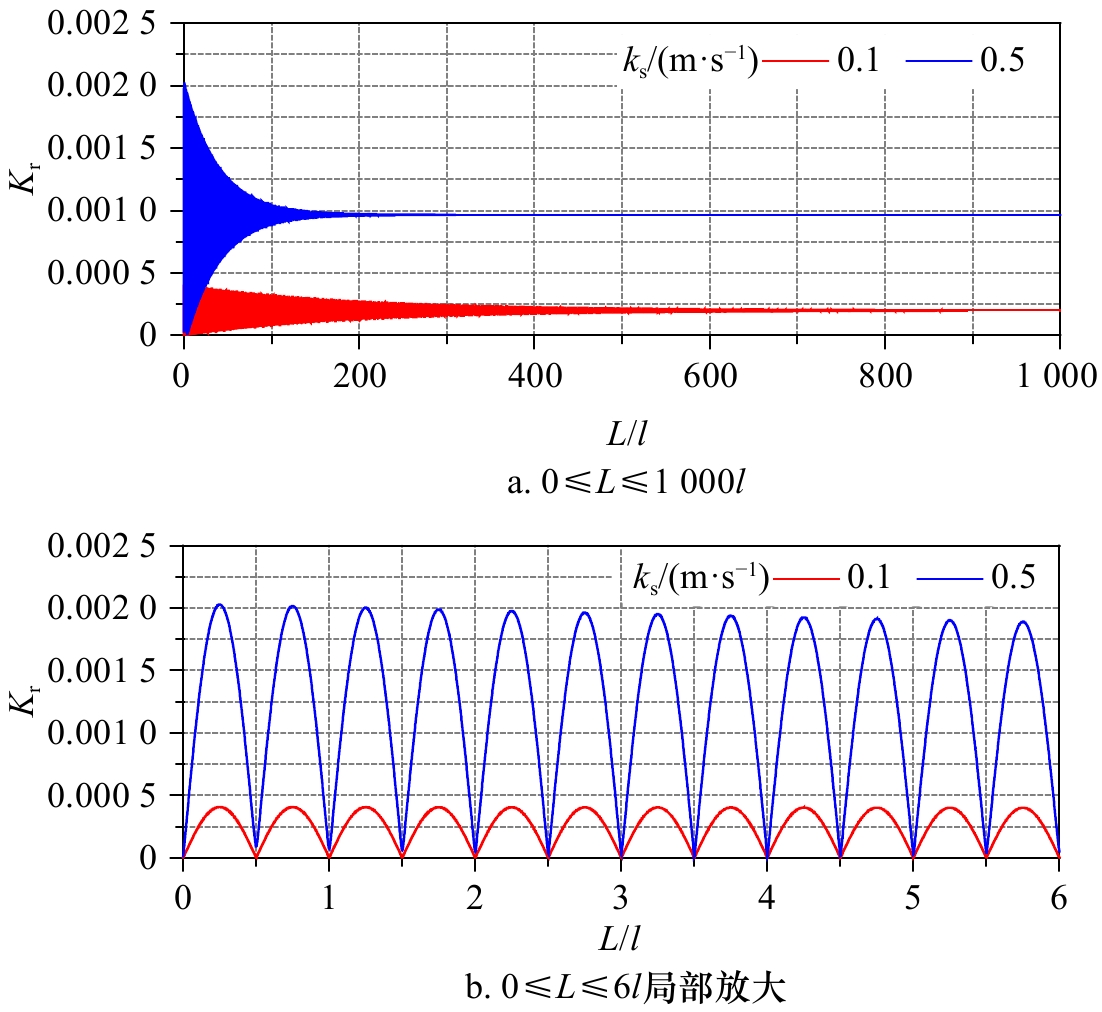
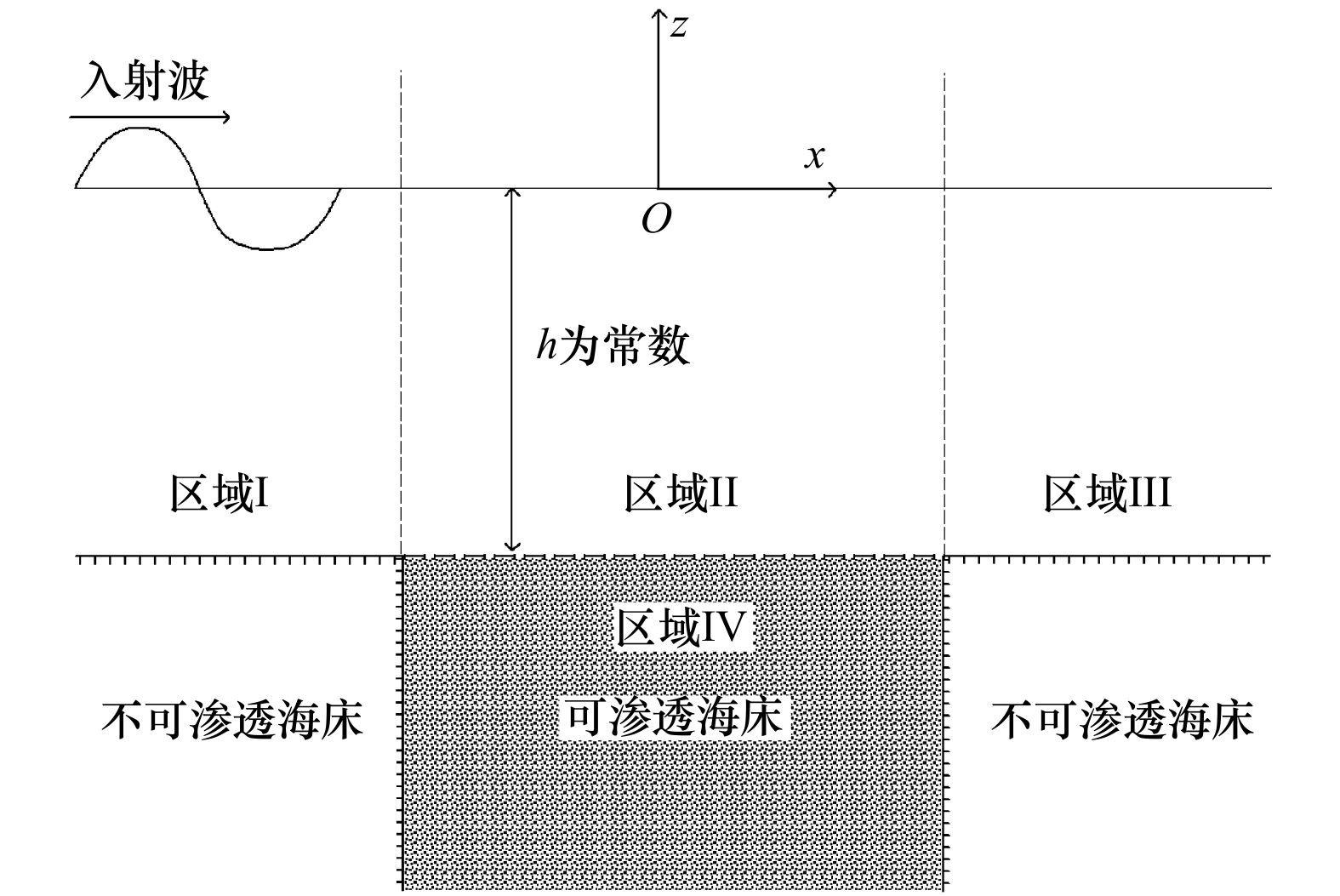
摘要: 本文建立了波浪在局部可渗透水平海床上传播的解析解，并研究了波浪在局部可渗透海床上的透射、反射问题。研究中将计算域划分为4个区域，中间区域为流域，海底可渗透，其下区域为多孔介质海床，左右两个区域也为流域，但海底不可渗透。应用线性波浪理论，建立了各流域包含非传播模态的速度势表达式，给出了海床内部的压强表达式，并利用交界面上匹配条件，求解了表达式中的待定系数。基于该解析模型，探讨了海床渗透系数、相对水深、渗透海床长度对波浪传播变形的影响。结果表明，波高沿程衰减，强度随渗透系数、渗透海床长度的增加以及相对水深的减小而变大；局部可渗透海床会引起波浪的反射和透射，随着海床长度的增加，反射系数振荡变化，并最终趋于常数，透射系数指数衰减，并最终趋于0。
- 局部渗透海床 /
- 非传播模态 /
- 复波数 /
- 反射系数 /
- 透射系数
Abstract: The present study is concerned with the analytical solution for waves propagating over a local permeable seabed and wave reflection and transmission by the local permeable seabed. The computational domain is decomposed into four subdomains of which the middle subdomain is permeable, with the porous seabed beneath it, and the left and right subdomains are impermeable. Applying the linear wave theory, the velocity potential of each fluid subdomain is set up, including the effect of evanescent mode, and the pressure inside the porous seabed is given. The unknowns are solved by the continuous conditions at the interfaces between the neighboring subdomains. The effect of permeability coefficient, water depth and length of permeable seabed on wave transformation is discussed. The results indicate the wave height attenuates increasingly with the increase of permeability coefficient, the length of permeable seabed, and decrease of water depth. Wave reflection and transmission will occur due to the local permeable seabed. The reflection coefficient oscillates, and tends to be constant eventually, while the transmission coefficient reduces exponentially, and tends to be zero with the increase in the length of permeable seabed.
- local permeable seabed /
- evanescent mode /
- complex wavenumber /
- reflection coefficient /
- transmission coefficient

HTML全文

图 1 波浪在局部可渗透海床上传播示意图

Fig. 1 Definition sketch of wave propagation over a local porous seabed

下载: 全尺寸图片幻灯片

图 2 动水压强截断项M取值分析

Fig. 2 Analysis of the value of the pore pressure truncation item M

下载: 全尺寸图片幻灯片

图 3 不同渗透系数情况下相对波高分布情况

Fig. 3 Wave height distribution for different permeability coefficients

下载: 全尺寸图片幻灯片

图 4 反射系数和透射系数随渗透系数变化情况（h/l=0.375，L=6l）

Fig. 4 The change of reflection coefficient and transmission coefficient with permeability coefficients (h/l=0.375, L=6l)

下载: 全尺寸图片幻灯片

图 5 不同相对水深情况下相对振幅分布情况

Fig. 5 Wave height distribution for different water depth

下载: 全尺寸图片幻灯片

图 6 反射系数和透射系数随相对水深变化情况（k_s=0.5 m/s，L=6l）

Fig. 6 The change of reflection coefficient and transmission coefficient with water depth (k_s=0.5 m/s, L=6l)

下载: 全尺寸图片幻灯片

图 7 反射系数随渗透海床相对长度变化情况

Fig. 7 The change of reflection coefficient with the length permeable seabed

下载: 全尺寸图片幻灯片

图 8 透射系数随渗透海床相对长度变化情况

Fig. 8 The change of transmission coefficient with the length permeable seabed

下载: 全尺寸图片幻灯片

表 1 不同渗透系数情况下复波数计算结果

Tab. 1 Complex wavenumber calculated for different permeability coefficients

水深h /m	h/l	波浪圆频率 ω/Hz	渗透系数k_s /(m·s⁻¹)	实部k_r /m⁻¹	虚部k_i /m⁻¹
15	0.375	1.229 6	0	0.157 08	0
15	0.375	1.229 6	0.05	0.157 08	0.000 03
15	0.375	1.229 6	0.08	0.157 08	0.000 05
15	0.375	1.229 6	0.20	0.157 07	0.000 13
15	0.375	1.229 6	0.50	0.157 06	0.000 32

下载: 导出CSV

表 2 不同相对水深情况下复波数计算结果

Tab. 2 Complex wavenumber calculated for different water depth

水深h /m	h/l	波浪圆频率 ω/Hz	渗透系数k_s /(m·s⁻¹)	实部k_r /m⁻¹	虚部k_i /m⁻¹
20	0.500	1.238 4	0.5	0.157 08	0.000 07
15	0.375	1.229 6	0.5	0.157 06	0.000 32
12	0.300	1.212 4	0.5	0.157 05	0.000 75

下载: 导出CSV

参考文献(19)

[1]	Savage R P, Fairchild J C. Laboratory study of wave energy losses by bottom friction and percolation[J]. Beach Erosion Board, 1953, 31: 1−25.
[2]	Özhan E, Shi-Igai H. On the development of solitary waves on a horizontal bed with friction[J]. Coastal Engineering, 1977, 1: 167−184. doi: 10.1016/0378-3839(77)90013-8
[3]	Gu Zhihao, Wang H. Gravity waves over porous bottoms[J]. Coastal Engineering, 1991, 15(5/6): 497−524.
[4]	Sawaragi T, Deguchi I. Waves on permeable layers[C]//Proceedings of the 23rd Coastal Engineering Conference. Venice: ASCE, 1992: 1531−1544.
[5]	Putnam J A. Loss of wave energy due to percolation in a permeable sea bottom[J]. Eos, Transactions American Geophysical Union, 1949, 30(3): 349−356. doi: 10.1029/TR030i003p00349
[6]	Reid R O, Kajiura K. On the damping of gravity waves over a permeable sea bed[J]. Eos, Transactions American Geophysical Union, 1957, 38(5): 662−666. doi: 10.1029/TR038i005p00662
[7]	Murray J D. Viscous damping of gravity waves over a permeable bed[J]. Journal of Geophysical Research, 1965, 70(10): 2325−2331. doi: 10.1029/JZ070i010p02325
[8]	Dean R G, Dalrymple R A. Water Wave Mechanics for Engineers and Scientists[M]. Singapore Teaneck, NJ: World Scientific, 1991.
[9]	Rojanakamthorn S, Isobe M, Watanabe A. Modeling of wave transformation on submerged breakwater[C]//22nd International Conference on Coastal Engineering. Virginia: ASCE, 1991: 1060−1073.
[10]	Silva R, Salles P, Palacio A. Linear waves propagating over a rapidly varying finite porous bed[J]. Coastal Engineering, 2002, 44(3): 239−260. doi: 10.1016/S0378-3839(01)00035-7
[11]	Tsai C P, Chen Hongbin, Lee F C. Wave transformation over submerged permeable breakwater on porous bottom[J]. Ocean Engineering, 2006, 33(11/12): 1623−1643.
[12]	Cruz E C, Isobe M, Watanabe A. Boussinesq equations for wave transformation on porous beds[J]. Coastal Engineering, 1997, 30(1/2): 125−156.
[13]	Méndez F J, Losada I J, Losada M A. Wave-induced mean magnitudes in permeable submerged breakwaters[J]. Journal of Waterway, Port, Coastal, and Ocean Engineering, 2001, 127(1): 7−15. doi: 10.1061/(ASCE)0733-950X(2001)127:1(7)
[14]	Huang C J, Chang H H, Hwung H H. Structural permeability effects on the interaction of a solitary wave and a submerged breakwater[J]. Coastal Engineering, 2003, 49(1/2): 1−24.
[15]	Mendez F J, Losada I J. A perturbation method to solve dispersion equations for water waves over dissipative media[J]. Coastal Engineering, 2004, 51(1): 81−89. doi: 10.1016/j.coastaleng.2003.12.007
[16]	Kirby J T, Dalrymple R A. Propagation of obliquely incident water waves over a trench[J]. Journal of Fluid Mechanics, 1983, 133: 47−63. doi: 10.1017/S0022112083001780
[17]	Bender C J, Dean R G. Wave transformation by two-dimensional bathymetric anomalies with sloped transitions[J]. Coastal Engineering, 2003, 50(1/2): 61−84.
[18]	宋岩新. 砂质海床上海底管线稳定性的数值分析[D]. 大连: 大连理工大学, 2008. Song Yanxin. Numerical analysis of stability of submarine pipelines on a sandy seabed[D]. Dalian: Dalian University of Technology, 2008.
[19]	钱琨, 王新志, 陈剑文, 等. 南海岛礁吹填钙质砂渗透特性试验研究[J]. 岩土力学, 2017, 38(6): 1557−1564, 1572. Qian Kun, Wang Xinzhi, Chen Jianwen, et al. Experimental study on permeability of calcareous sand for islands in the South China Sea[J]. Rock and Soil Mechanics, 2017, 38(6): 1557−1564, 1572.